Chapter 18 Probability Ex 18.1

RBSE Solutions for Class 10 Maths Chapter 18 Probability Ex 18.1

RBSE Board Class 10  Probability are part of  Maths Chapter 18. Here we have given Rajasthan Board RBSE Class 10 Maths Chapter 18 Probability Ex 18.1 in details. Hope this article will help you to understand Chapter 18 Probability Ex 18.1 in a better way.

Rajasthan Board RBSE Class 10 Maths Chapter 18 Probability Ex 18.1

Question 1.
Find the probability of getting a number grater than 4, when a dice is thrown once.
Solution:
When a dice is thrown once, the total possible outcomes (1, 2, 3, 4, 5, 6)
∴ Total number of all possible outcomes = 6.
The favourable outcomes of getting a number greater than 4 = 5, 6.
∴ The number of favourable outcomes = 2
∴ Required probability = \frac { 2 }{ 6 } = \frac { 1 }{ 3 }

Question 2.
A coin is tossed twice simultaneoeusly. Find the probability of getting a head on both times.
Solution :
When a coin is tossed twice. All possible outcomes are (H, H), (H, T), (T, H), (T, T)
∴ The total number of all possible out comes = 4
The favourable outcomes of getting heads twice = (H, H).
∴ Required probability = 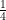\frac { 1 }{ 4 }

Question 3.
A number is drawn at random from the natural number 1 to 17. Find the probability that the number drawn is a prime.
Solution :
Total number of all possible outcomes of natural numbers 1 to 17 = 17
Prime numbers in 1 to 17 = 2, 3, 5, 7, 11, 13 and 17.
∴ Number of favourable outcomes = 7
∴ Required probability = \frac { 7 }{ 17 }

Question 4.
Find the probability of getting head or tail alternatively if a coin is tossed simultaneously three times.
Solution :
A coin is tossed three times.
∴ Total possible outcomes are HHH, HHT, HTH, THH, HTT, THT, TTH and TTT.
∴ Total number of all possible outcomes =8
The favourable outcomes of getting head to tail alternatively HTH, THT.
∴ The number of favourable outcomes = 2
∴ Required probability = \frac { 2 }{ 8 } = \frac { 1 }{ 4 }

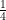
Question 5.
Find the probability of falling 52 Sundays in non-leap year.
Solution :
The non-leap year has 365 days in it. There are 52 weaks in a year i.e., 52 Sundays.
The remaining 1 day may be either, Sunday, Monday, Tuesday, Wednesday, Thursday, Friday and Saturday.
∴ Total number of possible outcomes = 7
The number of net falling Sunday = 6
∴ The probability of falling only 52 Sundays in a non-leap year = 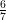\frac { 6 }{ 7 }

Question 6.
If P(A) = 0.65, then find the probability of “not A”.
Solution :
Given, P(A) = 0.65
∴ P(not A) = 1 – P(A)
= 1 – 0.65 = 0.35

Question 7.
Two coins are thrown at the same time. Find the probability that the top faces of coins will contain maximum two tails.
Solution :
Tossing two coins at the same time.
Total possible outcomes = HH, HT, TH, TT
∴ Total number of possible outcomes = 4
Favourable outcomes of getting maximum one tail are HT, TH and TT.
∴ Number of favourable outcomes = 3
∴ Required probability = 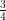\frac { 3 }{ 4 }

Question 8.
A dice is thrown twice. Find the probability that the sum of the digits on the top faces of dice is:
(i) 9
(ii) 13.
Solution :
When a coin is tossed twice, the digits on the top faces may be
(1, 1,) (1, 2), (1, 3),(1, 4), (1, 5), (1, 6), (2, 1), (2, 2), (2, 3), (2, 4),(2, 5), (2, 6), (3, 1 ),(3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (4, 1), (4, 2), (4, 3), (4, 4),(4, 5), (4, 6), (5, 1), (5, 2), (5, 3), (5,4), (5, 5), (5, 6), (6, 1), (6, 2), (6, 3), (6, 4), (6, 5), (6, 6).
∴ Total number of all possible outcomes = 36
(i) The favourable outcomes of getting the sum 9 = (6, 3), (5, 4), (4, 5), (3, 6)
The number of favourable outcomes = 4
∴ Required probability = \frac { 4 }{ 36 } = \frac { 1 }{ 9 }
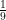
(ii) When the dice is thrown two times, then the favourable outcomes of getting a sum 13 = 0.
Hence the probability = 0.

Question 9.
A bag contains 5 red balls and 3 white balls. A ball is taking out at random from it. What is the probability that the ball is
(i) white
(ii) not white.
Solution :
Number of balls in the bag.
= 5 red + 3 white = 8
Getting out a ball from the bag at random, the total number of possible outcomes = 8
(i) The number of favourable outcomes of the ball drawn is white = 3
∴ The probability of the ball drawn is white P(W)
RBSE Solutions for Class 10 Maths Chapter 18 Probability Q.9
(ii) The probability that the ball is not white = P(\overline { W }) = 1 – P(W)
= 1 – \frac { 3 }{ 8 } = \frac { 8-3 }{ 8 } = \frac { 5 }{ 8 }
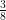Hence, (i) The probability that the ball is white = 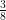\frac { 3 }{ 8 }
(ii) The probability that the ball is not white = \frac { 5 }{ 8 }

Question 10.
By chance 12 defected pens are mixed into 132 good pens. On seeing them it cannot be decided which pen is defected and which is good. If at random one pen is drawn out them, what is the probability that pen is good?
Solution :
The number of defected pens = 12
Number of good pens = 132
Total number of pens = 12 + 132 = 144
The probability that the pen drawn is good
The number of favourable outcomes of
RBSE Solutions for Class 10 Maths Chapter 18 Probability Q.10
= \frac { 132 }{ 144 } = \frac { 11 }{ 12 }
Hence, the probability that the pen drawn is good = \frac { 11 }{ 12 }

Question 11.
A card is drawn from a well shuffled deck of 52 playing cards. Find the probability of the following :
(i) A red jack
(ii) A red card
(iii) An ace of hearts
(iv) A queen of diamonds
(v) A card of spades.
Solution :
A deck of playing cards has 52 cards.
Shuffling the deck properly and one card is drawn, then the total possible outcomes = 52.
(i) There are four jack in the deck of which the jack of hearts arid diamonds are red.
∴ The number of favourable outcomes of drawing a red jack = 2
∴ Probability that the card drawn is a red jack = 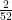\frac { 2 }{ 52 } = \frac { 1 }{ 26 }

(ii) The deck contains 13 cards of hearts and 13 cards of diamonds. So the number of favourable outcomes that the card is red = 26.
∴ The probability that the card drawn is red = 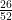\frac { 26 }{ 52 } = \frac { 1 }{ 2 }

(iii) There is only one hearts ace in the deck.
So, the number of favourable outcomes = 1
∴ The probability that the drawn card is a hearts ace = \frac { 1 }{ 52 }

(iv) There is only one diamonds queen in the deck, So, the number of favourable outcomes of getting a diamond queen = 1
∴ The probability that the card drawn is a diamonds queen = \frac { 1 }{ 52 }

(v) There are 13 spades cards in the deck so, the favourable outcomes = 13

∴ The probability that the card drawn is a spades = 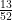\frac { 13 }{ 52 } = \frac { 1 }{ 4 }
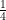
We hope that the material provided on this website will be helpful for you. If you have any query regarding any topic related to Rajasthan Board of Secondary education then drop a comment below we will try give you response as soon as possible. if you enjoyed the post,  i'd very grateful if you would help it spread by emailing it to a friend, or sharing it on twitter or facebook. Thank you!
shubham Jadoun

Posted by shubham Jadoun

Post a Comment

0 Comments