Chapter 19 Road Safety Education

RBSE Solutions for Class 10 Maths Chapter 19 Road Safety Education

Class 10 Maths Chapter 19 Road Safety Education Solution is provided in this post. Here we have provide the solutions of RBSE Boards Books according to chapter wise.

Rajasthan Board RBSE Class 10 Maths Chapter 19 Road Safety Education

Exercise

Question 1.

The distance between A and B is 150 km and there are ten (10) traffic signals in between A and B. If a car by 60 km/h speed reaches point B crossing all green signals in 2 hours and 30 minutes but on other days due lo heavy traffic it happens to stop as follows:

First Traffic Signal : 1 minute

Second Traffic Signal : 2 minutes

and up to tenth (10th) Signals : 10 minutes

Calculate the total time taken by that car if it follows the total traffic signals (excepts/baring other hurdles) while the speed of the car is 60

km per hour.

Solution :

According to question, we write the total time of stooping at the signals in the form of series, then 1, 2, 3, 4………..10.

a = 1, d = 2 – 1 = 1, n = 10

∴ Total time to be stopped at all signals.

St = [2a+(t-1)d]

⇒ St = 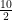[2 × 1 + (10 – 1)1]

⇒ St = 5[2 + 9]

⇒ St = 55 min

Hence, total time taken by car reaching from A to B

2 h 30 min + 55 min = 3 h 25 min

Hence, car will take 3 h 25 m to reach B in other days.

Additional Questions

Short/Long Answer Type Questions

Question 1.

Are all the numbers from t to 9 in arithmetic series ? Justify your answer.

Solution :

Numbers from 1 to 9 can be written series as following:

1, 2, 3, 4, 5, 6, 7, 8, 9

Thus,

a1 = 1,         a4 = 4,            a7 = 7

a2 = 2,         a5 = 5,            a8 = 8

a3 = 3,         a6 = 6,            a9 = 9

Common difference = d and it is AP.

∴ d = a2 – a1 = a3 – a2 = a4 – a3 = a5 – a4 = ……..

⇒ d = 2 – 1 = 3 – 2 = 4 – 3 = 5 – 4 =……

⇒ d = 1

Hence, numbers are in AP from 1 to 9 since it common difference is equal.

Question 2.

Find the first four terms of an A.P. formed by using first term, a = 2 and common difference, d = 3.

Solution :

a = 2, d = 3

∴ First term a1 = a = 2

Second term = a2 = a1 + d = 2 + 3 =5

Third term = a3 = a2 + d = 5 + 3 = 8

Fourth term = a4 = a3 + d = 8 +3 = 11

Hence, four terms of series are 2, 5, 8, 11.

Question 3.

If on a road from a stone, 11th signal and 16th signal are mounted at 380 m and 730 m respectively, then find the distance at which 31st signal is mounted.

Solution : an = a + (n – 1)d

From equation a11 = a + 10d = 380

∴ a + 10d = 380 ……(i)

a16 = a + 15d = 730

a + 15d = 730

Subtracting (i) from (ii)

(a + 15d) – (a + 10d) = 730 – 380

⇒ 5d = 350

⇒ d = 70

Putting the value of d in (i)

a + 10 × 70 = 380

⇒ a = 380 – 700

a = – 320

∴ Distance of 31st signals from stone.

a31 = a + 30d = -320 + 30 × 70

= -320 + 2100

= 1780 m

Question 4.

Complete the table:

Solution :

(i) a8 = a + 7d

⇒ a8 = 7 + 7 × 3 = 28

(ii) a10 = a + 9d

⇒ 0 = -18 + 9 × d

⇒ d = 2

(iii) a18 = a + 17d

⇒ -5 = a+ 17(-3)

⇒ a = 51 – 5 = 46

(iv) an = a + (n – 1)d

⇒ 3.6 = -18.9 + (n – 1)2.5

⇒ 2.5 (n – 1) = 22.5

⇒ (n – 1) =  = 9

⇒ n = 10

(v) a105 = a + 104d

⇒ a105 = 3.5 + 104 × 0

⇒ a105 = 3.5

Question 5.

To cars start to run from same place into same direction. Speed of first car is 100 km/h. Now,speed of second car is 8 km/h for Ist hour and its speed increases 0.5 km/h. If both cars run without any resistance, then find the time in which second car will cross the first car.

Solution :

Let, second car will cross first car in ‘n’ hours. Both cars will cover same distance n ‘n’ hours. First car will cover 10 n km distance where second car will cover

= Sn, where a = 8, d = 1/2

= (2 × 8 + (n – 1) × )

= 

When second car crosses first car, then

 = 10n

⇒ n + 31 = 40

⇒ n = 9

Hence, second car will cross first car in 9 hours.

Question 6.

Some vehicles are parked in no parking zone on a road, in which 10 m distance is maintained between each vehicles. A larger vehicle (truck) of municipality is standing in middle of the place. Crane can load one vehicle into larger truck in a single time. Total number of vehicles on the road is add excluding crane. At last crane covers a distance of 3 km. Find the total number of vehicle including crane.

Solution :

Let total vehicles on road excluding crane = 2n + 1. Vehicles on both sides of larger vehicle = n + n.

Distance covered in lifting vehicles by crane between P and M.

Sn = 10n + 2[10(n – 1) + 10(n – 2) + ………..+10 × 2 + 10 × 1]

Similar, Distance covered in lifting vechiles between Q and M.

Sn‘ = 2[10n + 10(n – 1) + 10(n – 2) + ………+10 × 2 + 10 × 1]

Total distance covered

S = Sn + Sn‘

∵ Since crane covered 3000 m (3 km) distance in total.

∴ 20n2 + 10n = 3000

⇒ 2n2 + n – 300 = 0

⇒ (n – 12)(2n + 25) = 0

∵ n ≠ -ive

n – 12 = 0

∴ 2n + 1 = 2 × 12 + 1 = 25

Hence, there, are total 26(25 + 1) vehicles including crane.

Compilation of Statistics

1. Datas are the groups, which are represented in numbers and affected by any uncalculated reasons upto a sufficient limit.
2. Datas are guessed according to purity of sufficient quantity. These are collected for a pre-define aim and represented in relation with each other.
3. In study of datas, a result is obtained and declared then.
4. Methods of representation of data are following:
   • Tabulation
   • Pie-graph
   • Bar graph etc.
5. It is necessary to control the pollution spread by vehicles. Emphasis is being exercised on the use of means of minimise pollution.

Exercise

Question 1.

See the following graph and discuss that in which year the level of main pollutant CO (Carbon monoxides) is minimum and to whom you can give its credit ? Why?

Solution :

The quantity of main pollutant (carbon monoxide) is minimum is the year 2003.

We can give its credit to the government because government introduced and encourage theuse of more efficient fuel which are low polluting such as CNG or LPG etc, stress given on PUC and increasing the awareness in the people about the environment and hazards of polluting the environment.

Additional Questions

Short/Long Answer type Questions

Question 1.

What as the importance of study of datas.

Solution :

It is used for forecasting of factors related to study.

Question 2.

How air pollution can be controlled by analyzing datas?

Solution :

Methods :

1. Display the quantity of pollution on a diagram by writing the polluting vehicles one factories in a group
2. Prepare a parameter (standard) with the concept of safe atmosphere.
3. Then after examine all
4. If a vehicle or a factory discharges pollution more than the parameters accepted, then start legal processing (action) against defectors, pollution can be reduced by counselling their silence (s).

Question 3.

Do you know that P.U.C. is compulsory for each vehicle ? What steps are taken in favour of PUC ?

Solution : Format of PUC

P.U.C. is compulsory for all vehicles. Government has introduced LP.G. (Liquified Petrolium Gas) and C.N.G (Compressed Natural Gas) instead of petrol.

Question 4.

The following pie-chart shows the distribution of various vehicles during the pollution checking campaign by a candidate. Answer the following question based on this pie-chart.

If total pollution is 600 unit, then

(i) Find the pollution done by car.

(ii) How much more pollution done by truck in comparion with school vehicles.

(iii) Find the difference in pollution done by car and bikes.

Solution :

(i) Pollution done by cars =  ×  = 175 unit

(ii) Difference in pollution done by school vehicles and trucks

=  =  ×  unit = 150 unit

(iii) Difference in poihition done by cars and bikes

=  =  ×  unit = 100 unit

Question 5.

Percentage of number of accidents and number of deaths in accidents. is shown graph given below :

Answer the following :

(i) In which year, number of deaths are minimum.

(ii) Find the difference in number of accidents in 2010 – 2011.

(iii) Find the number of deaths in year 2012.

(iv) Find the number of alines in year 2012.

Solution:

(ii) Difference = 30000 – 20000 = 10000

Required percentage =  × 100%

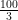= % = 33%

(iii) Number of deaths in 2010.

= 30000 ×  = 6750

(iv) Number of accidents in 2012 = 35000

Number of deaths = 35000 × 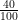 = 14000

Number of alives = 35,000 – 14,000 = 21,000

Application of Trigonometry

1. Shape bounded by three sides is known as triangle.

2. Measurement and study of triangle is known as trigonometry.

3. It ¡s based on Pythagoras theorem.

4. Ratios of sides of triangle is knows as trigonometric ratios.

5. Main ratios are following:

sin θ =  tan θ = 

cos θ =  cot θ = 

6. Application of trigonometry in respect of increasing of traffic and road accidents.

7. Since height and distance are used in measuring the height and distance of towers and buildings. It can also be used in day by day increasing road traffic and road accidents.

Exercise

Question 1.

A CCTV camera has to be installed on the top of a straight pole of 12 m height, so that traffic could be seen upto distance more than LOS of 13 m. Answer the following

1. What will be the distance from the foot of the pole, ahead of which traffic is seen ?

2. What will be area of the Green Patch around the pole ?

3. Do you think that CCTV camera is useful in the management of traffic awareness ? If yes, then how ?

Solution :

The minimum distance between the feet of the pole and vehicle will be 5 m.

2. Area of the circle around the pole which can not be observed by the CCTV camera

= πR2

= 3.14 × (5)2

= 78.5 m2

Yes, CCTV camera s useful manage and control the traffic.

Following are the facts in favor of the above statement:

(i)All the drivers of the vehicles become alert, by fear of the challan.

(ii) No, driver can shift their blames on others.

(iii) The accidents reduces because all the drivers tey to drive safely.

(iv) The traffic police become more efficient.

Additional Questions

Short/Long Answer Type Questions

Question 1.

A camera is mounted on a wall (AB) in the middle of a crossing. Its end B ¡s in contact with earth (road). Let the focus point of camera is C on earth which Is 3 m far from B. Find the horizontal distance between camera and point C.

(Given : ∠ACB = 60°)

Solution:

Question 2.

Some small guns are mounted at the top of a police van which make an angle 30° with horizontal for security purpose. If a muzzle fired from gun covered a distance of 184 m, then find the height of last point of distance covered by muzzle with surface of earth.

Solution :

sin θ =  (Formula)

⇒ AC = AB sin θ

⇒ AC = 184 sin 30°

⇒ AC = 184 × 

⇒ AC = 92 m

Question 3.

Angle of depression changes from 60° and 45° of rotational camera. Visible region of camera ¡s now decreased by 45 m. Find the height at which camera is mounted.

Solution :

BC = 61.48 m

∴ AB = 61.48 m

Question 4.

The police were searching for some thieves with the help of searchlight in a dark ball. The police on first floor hear, noise on the ground floor. The police focused the search light and found that the shadow and the length of the thief, were equal :

(i) Find out the depression angle

(ii) Find this angle in gradually increasing, then does it mean that the shadow is distancing from the police? If the answer is yes, then clarify it.

Solution :

Let, police is A and thief is C then

tan θ = 

∵ AB = BC

⇒ tan θ =  = 1 = tan 45°

Thus θ = 45°

If this angle is increasing, then it means thief is going far away from police and police can never catch thief.

Hence police can not catch thief ever.

Question 5.

The length of two poles is 20 m and 14 m respectively. Both the poles one connected with a wire. If wire makes 300 angle of elevation with horizontal, then find the length of wire.

Solution :

tan θ = , Let PR is wire

⇒ tan θ = 

PR = 12 m

Hence length of wire is 12 m

Question 6.

An electrician wants to repair the electric fault on the electric pole (5 m high or height 5 m) He has to reach 1.3 m downward from the ultimum point of the pole. If a ladder is put at angle from the horizontal, then find out its length. Also state the distance of the pale from the ladder.

Solution :

⇒ Distance of pole from ladder, CD = 2.14 m

Length of Staire (BD)

Problems based on two variable quantities

1. Variable quantities stains different values in different situations., e.g., x, y, z, a, b, e, p, q, r etc.

2. When any problem depends on different factors, then to solve these we need concept of linear equations and methods to solve it.

3. Obtained values are the solution of problems.

4. Methods of solve linear equations are following:

1. Companion method
2. substitution method
3. Elimination method
4. Cross-multiplication method
5. Ratio-decision method
6. Graphical method.

5. Standard from of linear equation is :

ax + by + c = O

and a1x + b1y + c1 = 0

where a, b, c, a1, b1, c1 = fixed quantities

x, y, z = variable quantity.

6. Relation an between stopping distance and velocity

s =  ⇒ s ∝ u2

7. Reaction Distance : Distance in which we react to stop our vehicle.

8. Breaking Distance : Distance in which we stop our vehicle after reason.

9. Stopping Distance : It is sum of reaction and breaking distance.

Reaction Distance + Breaking Distance = Stopping Distance

Exercise

Question 1.

A car moves with a speed of 50 km/h. If stopping distance ¡s 40 m and its rate of retardation is 4.4 m/s2, then find the time of arrival.

Solution :

Speed of car

Velocity (u) 50km/h = 50 × =  = 

u = m/s

Stopping distance (s) = 40m

Retardation (a) = + 4.4 m/s2

Time of reaching (t) = ?

From equation of motion :

v = u – at

⇒ 0 =  – 4.4t

t = 

t = 3.16 s

Hence, car can be stopped in 3 sec.

Question 2.

Complete the table :

Solution :

Total stopping distance = Reaction distance × Chasing distance

(i) 18 = 9 × 2

(ii) 54 = 18 × __

Question 3.

See the following formula :

Stopping distance = (Distance of reaction) + (Breaking Distance)

(i) Do the stopping distance changes according to the speed of the vehicle ?

(ii) How does it changes if the road is wet and slippery?

Solution :

(i) Yes.

(ii) It increases on the wet and slippery road.

If two similar vehicles are running with same speed, one vehicle in running on dyer and non slippery road then its stopping distance will less

comparative to the vehicle running on wet and slip prey road.

Additional Questions

Question 1.

In a bus, the cost of 2 tickets for stoppage A and 3 tickets for stoppage B is ₹ 46, whereas the cost of 3 tickets for stoppage A and 5 tickets for stoppage B is ₹ 74. Find the fare from stoppage A to B.

Solution :

Let Fare of A = ₹ x and fare of B = ₹ y, then

2x + 3y = 46

3x + 5y = 74

Multiply by 3 in eq. (i) and by 2 in eq. (ii) and then subtracted (ii) from (i).

6x + 9y – 6x – 10y = 138 – 148

⇒ -y = -10

⇒ y = 10

putting y = 10 in equ. (i)

2x + 3 × 10 = 46

x =  = 8

x = 8

Hence, fare of A ₹ 8, fare of B = ₹ 10.

Question 2.

A car running with speed 10 m/s can be stopped in 20 m on applying a constant force. If its speed were 30 mIs, then find the minimum distance in which it can be stopped by applying the same force.

Solution :

Let it can be stopped in distance ‘S2’. then

u1 = 10 m/s

u2 = 30 m/s

S1 = 20 m

S2 = ?

∵ S ∝ u2

⇒ S2 = 20 × 9

⇒ S2 = 180 m

Question 3.

In a city, a fixed charge and charge for a distance Is included in the fare. If a passenger pays ₹ 105 for 10 km and ₹ 155 for 15 km,then find the fixed charge and charge per km.

Solution:

Let fixed charge = ₹ x

Charge for distance = ₹ y per km

them according to question

x + 10y = 105 ….(i)

x + 15y = 155 …(ii)

on subtraction (i) from (ii)

5y = 50

⇒ y = 10

from equation (i)

x + 10(10) = 105

⇒ x + 100 = 5 + 100

⇒ x = 5

Hence, fixed charge is ₹ 10 and charge for distance is ₹ 5 per km.

Question 4.

Two places A and B are at 6 km distance from each other. At he same time two people start to run towards each other and meet in 45 mm. If they run in same direction, then they meet in 6 hours. Find their speed.

Solution :

Let their speed be x and y km/h them

x + y =  = 8

⇒ x + y = 8

⇒ x – y =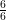 

⇒ x – y = 1

on solving (i) and (ii)

2x = 9, x = 4.5 km/h

and y = 3.5 km/h

Hence, their spiced are 4.5 km/h and 3.5 km/h

Question 5.

Hema travels the distance of 300 km through train as well as by bus for reaching home. She arrives home with in 4 hrs. She travels upto 60 km through train and the rest of the distance cornered by bus. If she travels 100 km through train and the rest by bus, it takes 10 minutes more. Find the speed of the train and the lens.

Solution :

Let, speed of train is x km/h and speed of bus y km/h then time taken by train = h

and time taken by bus = h

According to question

Hence, speed of train is 60km/h and of bus 80 km/h.

We hope that the material provided on this website will be helpful for you. If you have any query regarding any topic related to Rajasthan Board of Secondary education then drop a comment below we will try give you response as soon as possible. if you enjoyed the post,  i'd very grateful if you would help it spread by emailing it to a friend, or sharing it on twitter or facebook. Thank you!