Chapter 4 Text Book Questions
RBSE Class 12 Chemistry Chapter 4 Multiple Choice Questions
Question 1.
The unit of rate constant of zero order reaction will be
(a) mol L-1 s-1
(b) L mol-1 s-1
(c) s-1
(d) mol2 L-1 s-1
Question 2.
The half life of the first order reaction is 69.35, then its rate constant will be
(a) 10-2 s-1
(b) 10-4 s-1
(c) 10 s-1
(d) 102 s-1
Question 3.
The rate constant of reaction is 7.239 x 10-4 s-1, then the order of reaction will be
(a) 0
(b) 1
(c) 2
(d) 3
Question 4.
Which the following statement is true for the first order reaction?
(a) The rate of reaction is directly proportional to zera power of concentration of reactants.
(b) The unit of rate constant is mol L-1 s-1.
(c) The half life of reaction does not depend on initial concentration of reactants.
(d) Can not be say directly.
Question 5.
On plotting a graph between log K and 1/T for first order reaction. a straight line is obtained. The slope of line will be
(a) – 
(b) – 
(c) – 
(d) – 
Question 6.
The rate of reaction increases rapidly on slightly increase in temperature, because
(a) The number of activated reactants increases.
(b) The number of collisions increases.
(c) The number of free path increases.
(d) Heat of reaction increases.
Question 7.
Which of the following relationship is correct for zero order reaction?
(a) t3/4 = t1/2
(b) t3/4 = 1.5 t1/2
(c) t3/4 = 0.25t1/2
(d) t3/4 =  t1/2
t1/2
Question 8.
Arrhenius equation is :
(a) k = – A e-Ea/RT
(b) k = A e-Ea/RT
(c) k = A e Ea/RT
(d) k = e-Ea/RT
Question 9.
The half life of first order reaction is 480s. Then rate constant will be
(a) 1.44 x 10-3 s-1
(b) 1.44 s-1
(c) 0.72 x 10-3 s-1
(d) 2.88 x 10-3 s-1
Question 10.
Time required to complete 90% first order reaction will be
(a) 1.1 times of half life
(b) 2.2 times of half life
(C) 3.3 times of half life
(d) 4.4 times of half life
Answers:
(a) 2. (a) 3. (b) 4. (c) 5.(b) 6. (a) 7. (b) 8. (b) 9. (a) 10. (c)
RBSE Class 12 Chemistry Chapter 4 Very Short Answer Type Questions
Question 1.
The rate law for a reaction A + B → product, is given by ; r = k [A] [B]2. What is the order of reaction?
[B]2. What is the order of reaction?
Solution:
Order of reaction =  =
=  or 2.5
or 2.5
Question 2.
The transformation of the molecule X into Y follows second order kinetics. If the concentration of X increases to three times, how will it affect the rate of formation of Y ?
Solution:
According to question, r1 = k[X]2 …(i)
If concentration is increased to three times, then
r2 = k [3X]2
r2 = k 9 X2
r2 = 9k [X]2 …(ii)
Divide eq (ii) by eq. (i)
r2 = 9 x rı
i.e., if concentration is increased there times, the rate increases 9 times.
RBSE Class 12 Chemistry Chapter 4 Short Answer Type Questions
Question 1.
For the reaction R P, the concentration of a reactant changes from 0.03 to 0.22 M in 25 minutes. Calculate the average rate of reaction using units of time both in minutes and seconds.
Solution:
Average rate =

Average rate = 4 x 10-4 mol L-1 min-1
= 6.66 x 10-6 mol L-1 s-1
Hence average rate = 4 x 10-4 mol L-1 min-1
Average rate = 6.66 x 10-6 mol L-1 s-1
Question 2.
In a reaction. 2A → Products, the concentration of A decreases from 0.5 mol L-1 to 0.4 mol L-1 in 10 minutes. Calculate the rate during this interval.
Answer:
For, the reaction,
2A → products

Question 3.
The first order reaction has a rate constant 115 x 10-3 s-1 How long will 5g of this reactant take to reduce to 3g?
Solution:
For first order reaction,

t = 0.444 x 103
t = 444 s
Question 4.
Time required to decompose SO2Cl2 to half of its initial amount is 60 minutes. If the decomposition is of first order reaction, calculate the rate constant for the reaction.
Solution:
t1/2 = 60 min
For first order reaction,
k = 
= 
k = 0.01155 min-1 = 1.155 x 10-2
k = 1.925 x 10-4 s-1
Question 5.
What will be the effect of temperature on rate constant?
Solution:
Rate constant of reaction is nearly doubled with rise in temperature by 10°C. The exact dependance of the rate constant on temperature is given by Arrhenius equation k = Ae-Ea/RT where A is called frequency factor and Ea is the activation evergy of the reaction.
T = temperature
R = gas constant.
Question 6.
The rate of the chemical reaction doubles for an increase of 10K from 298 K. Calculate Ea
Solution:
Temperature T1 = 298K
Temperature T2 = 308K
Rate constant k2 = 2 x k1

= 52897.78 J mol
Ea = 52.9 kJ / mol
Question 7.
The activation energy for the reaction 2HI(g) → H2(g) + 12(g) is 209.5 kJ mol-1 at 581 K. Calculate the fraction of molecules of reactants having energy equal to or greater
than activation energy.
Solution:
Fraction of molecules having energy equal to or greater than activation energy

x = Antilog (- 18.8323)
= Antilog 19.1677
x = 1.471 x 10-19
Question 8.
From the rate expression for the following reactions, determine their order of reaction and the dimensions of the rate constants.
(i) 3NO(g) → N2O(g) + NO2(g); Rate = k [NO]2
(ii) H2O2(ap) + 3I–(aq) + 2H+ → 2H2O(l) +  ; Rate = k[H2O2] [I–]
; Rate = k[H2O2] [I–]
(iii) CH3CHO(g) + CH4(g) + CO(g) ; Rate = k [CH3CHO]
(iv) C2H5Cl(g) → C2H4(g) + HCl(g) ; Rate = k[C2H5Cl]
Solution:
(i) Rate = k [NO]2
Order of reaction = 2
Unit of rate constant = (mol L-1)1-n x s-1
n = order of reaction = (mol L-1)1-2 x s-1 = L mol-1 s-1
Hence order of above reaction is 2 and its unit is L mol-1 s-1.
(ii) Rate = k [H2Og][I–]
Order of reaction = 1 + 1 = 2
Unit of rate constant = (mol L-1)1 – n x s-1
= (mol L-1)1 – 2 x s-1
= (mol L-1) x s-1 = L mol-1 x s-1
For the reaction order is 2 and unit of rate constant is L mol-1 s-1
(iii) Rate = k (CH3CHO)
Order of reaction= 
Unit of rate constant = (mol L-1)1 – 15 x s-1 = mol-1/2 L1/2 s-1
(iv) Rate = k [C2H5CI]
Order of reaction = 1
Unit of rate constant = (mol L-1)1 – n x s-1 = s-1
Order is 1 and unit is s-1
Question 9.
For the reaction 2A + B → A2B, rate = k [A] [B]2 with k = 20 x 10-6 mol-2 L2 s-1. Calculate the initial rate of the reaction when [A] = 0.1 mol L-1 and [B] = 0.2 mol L-1 Calculate the rate of reaction after [A] is reduced to 0.06 mol L-1.
Solution:
Initial rate = k [A] [B]2
= 20 x 10-6 x 0.1 x (0.2)2
= 8.0 x 10-9 mol L-1
When concentration of [A] is reduced from 0.10 mol L-1 to 0.06 mol L-1 i.e., 0.04 mol L-1 concentration of A is used then used concentration of B is,
= 0.02 mol L-1
Hence [B] = 0.2 – 0.02
= 0.18 mol L-1
Rate = k [A] [B]2
Rate of reaction = 2.0 x 10-6 x 0.06 x (0.18)2
= 3.89 x 10-9 mol L-1 s-1
Question 10.
The decomposition of NH3 on platinum surface is a zero order reaction. What will be the rate of production of N2 and H2 when the value of k is 25 x 10-4 mol L-1 s-1?
Solution:
2 NH3 → N2 + 3H2
Rate of reaction =  (Decrease in concentration of NH3)
(Decrease in concentration of NH3)
= (Rate of formation of N2)
= 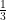 (Rate of formation of H2)
(Rate of formation of H2)

As the order of reaction is zero hence rate of reaction
= k [NH3]0
= 2.45 x 10-4 mol L-1 s-1
Rate of formation of N2
Rate of reaction = Rate of formation of N2
= 2.5 x 10-4 mol L s-1
Rate of formation of H2
Rate of reaction = 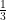 (Rate of formation of H2)
(Rate of formation of H2)
Rate of formation of H2 = 3 x Rate of reaction
= 3 x 2.5 x 10-4
= 7.5 x 10-4 mol L-1 s-1
Question 11.
Mention the factors that affect the rate of a chemical reaction.
Solution:
The factors that affect the rate of a chemical reaction are –
- Concentration :On increasing concentration of reactants, the probability of colloisions of molecules increases hence rate of reaction increases.
- Temperature :On increasing temperature, the kinetic energy of molecules increases, hence, the number of collision increases. Therefore, the rate of reaction also increases.
- Pressure :On increasing pressure, the molecules of gases come eloser to each other. As a result their collisions increase and hence rate of reaction increases.
- Surface Area of Reactants :On increasing surface area of reactants, the rate of reaction increases. For example, the powdered metals react faster than the metals in a lump.
- Nature of reactants :If the reactants are ionic in nature than the rate of reaction is faster than those in which reactants are molecular in nature.
Question 12.
A reaction is second order with respect to a reactant. How is the rate of reaction affected if the concentration of the reactant is
(i) doubled
(ii) reduced to  ?
?
Solution:
Rate = k [A]2 If concentration = a then,
Rate = k (a)2
(i) If concentration is doubled then,
[A] = 2a
(rate)1 = k (a)2
(rate)2 = k (2a)2
hence
(rate)2 = 4 x (rate)1
i.e., the rate becomes four times.
(ii) If concentration is reduced to half
[A] = 

i.e., rate becomes one fourth.
Question 13.
Following data were obtained for the hydrolysis of ester in water of pseudo order of reaction.
| Time (in sec) | 0 | 30 | 60 | 90 |
| Ester (mol L-1) | 0.55 | 0.31 | 0.17 | 0.0.85 |
(i) Calculate the average rate of reaction between the time interval 30 to 60 seconds.
(ii) Calculate the pseudo first order rate constant for the hydrolysis of ester.
Solution:
(i) Average rate during the interval 30 – 60 sec
= 4.67 x 10-3 mol L-1 s-1
(ii) Rate constant for pseudo first order reaction


= 1.98 x 10-2 s-1
Hene rate constant is 1.98 x 10-2 s-1
Question 14.
In a reaction between A and B, the initial rate of reaction was measured for different initial concontration of A and B as given below.
| A (mol L-1) | 0.20 | 0.20 | 0.40 |
| B (mol L-1) | 0.30 | 0.10 | 0.05 |
| r (mol L-1 s-1) | 5.07 x 10 | 5.10 x 10 | 1.43 x 10 |
What is the order of reaction with respect to A and B?
Solution:
Let the order of reaction w.r.t A is x and w.r.t Bis y.
Then rate law of reaction can be written as
Rate = k[A]x [B]y
With the help of given
(r0)1 = 5.07 x 10-5 = k[0.20]x [0.30]y …(i)
(r0)2 = 5.07 x 10-5 = k[0.20]x [0.10]y ….(ii)
(r0)3 = 1.43 x 10-4 = k(0.40)x [0.05]y. …(iii)
Dividing eq. (i) by (i) we get

1= (3)y
(3)0 = (3)y
∴y=0
Diving eq. (iii) by eq. (ii),

y = 0, So, 
Taking logarithm on both sides,
log 2.820 = x log 2
0.4503 = x × 0.3010
or
x =  = 1.5
= 1.5
∵ Rate = k[A]x [B]y
∴ Rate = k[A]1.5 [B]0
∴ Order of reaction = 1.5 + 0 = 1.5
Hence, order of reaction with respect to Ais 1.5 and with respect to B is 0.
Question 15.
The following results have been obtained during the kinetic studies of the reaction. 2A + B → C + D
| Experiment | [A] mol L-1 | [B] mol L-1 | Initial rate of formation of D (molL1min1) |
| I | 0.1 | 0.1 | 6.00 x 10-3 |
| II | 0.3 | 0.2 | 7.20 x 10-2 |
| III | 0.3 | 0.4 | 2.88 x 10-1 |
| IV | 0.4 | 0.5 | 2.40 x-10-2 |
What is the rate law ? what is the order with respect to each reactant and the overall order ? Also calculate rate constant and write its unit.
Solution:
Let the equation is,
Rate = k [A]x [B]y
Then According to given datas.
(rate)I = 6.0 x 10-3 = k(0.1)x (0.1)y.
(rate)II = 7.2 x 10-2 = k(0.3)x (0.2)y
(rate)III = 2.88 x 10-1 = k(0.3)x (0.4)y
(rate)IV = 2.40 x 10-2 = k(0.4)x (0.1) y
From equation (2) and (3)

y = 2
From equation (1) and (4)

x=1
Hence, rate = k [A] [B]2
because x = 1, y = 2.
Rate law is
rate = k [A] [B]2
Hence calculation of rate constant with the help of eq (1)
rate = k [A] [B]2
6.0 x 10-3 = k(0.1)(0.1)2
k = 
k = 6.0 mol-2 L2 min-1.
Question 16.
A reaction between A and B is first order w.r.t. A and zero order w.r.t. B. Fill in the blanks in the following table.
| Experiment | [A] /mol L“1 | [B] /mol L“1 | Initial rate / M min-1 |
| I | 01 | 0.1 | 2.0 x 10-2 |
| II | – | 0.2 | 40 x 10-2 |
| HI | 0.4 | 0.4 | – |
| IV | – | 0.2 | 2.0 x 10-2 |
Solution:
Rate law for the reaction is given as
Rate = k [A]1 [B]0 = k[A]
From experiment 1, we get
20 x 10-2 mol L-1 min-1 = k [0.1 mol L-1]

(i) In experiment II
Rate = k[A]
[A] = 

(ii) In experiment III
Rate = k[A]
= 0.2 min-1 x 0.4 M
= 0.08 M min-1 = 8.0 x 10-2 M min-1
(iii) In experiment IV
Rate = k[A]
[A] = 

Question 17.
Calculate the half life of first order reaction from their rate constants given below:
(a) 200 s-1
(b) 2 min-1
(c) 4 year-1
Solution:
For first order reaction,
Half life = 
(i) t1/2 =  = 3.465 x 10-3 s
= 3.465 x 10-3 s
(ii) t1/2 = 0.3465 min
(iii) t1/2 =  = 0.1732 year
= 0.1732 year
Question 18.
The half life for radioactive decay of 14C is 5730. yr. An archaelogical archetect contained wood that had only 80% of the 14C found in living tree. Estimate the age of the
sample.
Solution:
Radioactive decay follows first order kinetics,
Decay constant (k) = 
According to question, living tree has 80% 14C hence,

t =1845 years
Hence the age of sample is = 1845 years
Question 19.
The rate constant for first order reaction is 60 s-1 How much time will be required to decompose  part of initial concentration of reactant?
part of initial concentration of reactant?
Solution:
For the first order reaction,


t = 4.62 x 10-2 s.
Question 20.
During nuclear explosion, one of the products is 90 Sr with half life of 28.1 years. If 1 µg of 70 Sr was absorbed in bones of a newly born baby instead of calcium, how much of it will remain after 10 year, and 60 years. If it is not lost metabolically?
Solution:
Decay constant (k) = 
0.025 = 25 x 10-2 year-1
After 10 years, the left amount will be
[A]0 = 1 µg,
[A] = ?
t = 10 years
k = 2.5 x 10-2 year-1

log [A] = – 0.1086
[A] = Antilog (- 0.1086)
[A] = 0.78 µg
Hence after 10 years only 0.78 µg will remain.
Calculation of amount left after 60 years.
t= 60 years
[A]0 = 1 µg
k= 2.5 x 10-2 year-1
[A] = ?

– log [A] = 65.13 x 10-2
– log [A] = 0.6513
[A] = Antilog (-0.6513)
[A] = 0.2232 µg.
After 60 years only 0.2235 µg will remain.
Question 21.
For a first order reaction, show that the time required for 99% completion of a first order reaction is twice the time required for the completion of 90%.
Solution:
Time required for the completion of 99% reaction,
 ……(1)
……(1)Time required for the completion of 90% reaction,
 …… (2)
…… (2)Divide eq. (1) eq. (2)

t99% = 2 x t90%
Question 22.
A first order reaction takes 40 min for 30% decomposition. Calculate t1/2.
Solution:


Question 23.
For the decompostion of azoisopropane to hexane and nitrogen at 543 K, the following data is obtained
| t (sec) | p ( mm of Hg) |
| 0 | 35.0 |
| 360 | 54.0 |
| 720 | 63.0 |
Calculate the rate constant.
Solution:
(CH3)2 CHN = NCH(CH3)2(g) → N2(g) + C6H14(g)
Suppose pi is the initial pressure at t = 0
After time t say x mole of azoisopropane decomposes and the pressure is p.
(CH3)2 CHN = NCH(CH3)2 → N2 + C6H4
Intial pressure (at t = 0) Pi 0 0
pressure at time = t Pi – x x x
The rate constant at 360 s,
Pi = 35.0 mm
At time t, p = pi – x + x + x = Pi + x
x = p – Pi = 54 – 35 = 19.0 mm
Now p at time 360s = 35 – 19.0 = 16 mm
= 2.17 x 10-3 s-1
Similarly x = p- pi = 63 – 35 = 28 mm
p at time 720 sec = 35 – 28 = 7 mm
= 2.235 x 10-3 s-1
Question 24.
The following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume.
SO2Cl2(g) → SO2(g) + Cl2(g).
| Experiment | Time/ s | Total pressure/ atm |
| 1 | 0 | 0.5 |
| 2 | 100 | 0.6 |
Calculate the rate of reaction when total pressure is 0.65 atm.
Solution:
SO2Cl2(g) → SO2(g) + Cl2(g)

Total pressure after time ‘t
Pt = (P0 – x) + x + x
Pt = P0 + x
x = Pt – P0
Initial pressure = P0
Final pressure = P0 – x
= P0 – (Pt – P0)
= 2P0 – Pt

k=2.2316 x 10-3s-1
Pt = 0.65 atm
i.e., (P0 + P)= 0.65 atm
P=0.65 – P0 = 0.65 – 0.50
P= 0.15 atm
Pressure of SO2Cl2 at time t
PSO2Cl2 = P0 – P
= 0.50 – 0.15
= 0.35 atm
At time ‘t
Rate = k x PSO2Cl2
= 2.2316 x 10-3 x 0.35
= 7.8 x 10-4 atm s-1
Hence when total pressure is 0.65 atm then the rate will be 7.8 x 10-4 atm s-1
Question 25.
The rate constant for the decompositon of N2O5 at various temperature is given below.
| To/c | a | 20 | 40 | 60 | 80 |
| 10-5 x klis-1 | 0.0787 | 1.70 | 25.7 | 178 | 2140 |
Draw a graph between In k and  and calculate the values of A and Ea. Predict the rate constant at 30°C and 50°C
and calculate the values of A and Ea. Predict the rate constant at 30°C and 50°C
Solution:
For plotting curve between log k and  , we have to prepare this table.
, we have to prepare this table.
| T (K) | 273 | 293 | 313 | 333 | 353 |
| 0.003663 | 0.003413 | 0.003195 | 0.003003 | 0.002833 | |
| Ks-1 | 0.0787 x 10-5 | 1.70 x 10-5 | 25.7 x 10-5 | 178 x 10″5 | 2140 x 10-5 |
| logk | – 6.1040 | – 47696 | – 3.5901 | – 27496 | – 1.6996 |
A curve plotted with the help of above values is as follows.


= 97772.64J mol-1
= 97.772 kJ mol-1
We know that,

Compare the above equation with y = mx + c then,
log A = C at ‘y’ axis i.e., value of k on axis.
log A = ( -1+ 7.2) = 6.2
y2 – y1 = – ( -7.2)
Frequency (A) factor = antilog 6.2
= 1585000
= 1.585 x 106 collisions s-1
Value of k can be calculated with the help of curve.
| T | 1/T | Value of log k from graph | Value of k |
| 303 K | 0.003300 | – 4.2 | 6.31 x 10-5 s-1 |
| 232 K | 0.003096 | – 2.8 | 1.585 x 10-2 s-1 |
Question 26.
The rate constant for the decomposition of a hydrocarbon is 2.418 x 10-5 s-1 at 546 K. If the energy of activation is 179.9 kJ/mol, what will be the value of pre-exponential factor? Solution:
k = 2.418 x 10-5 s-1
Ea = 179.9 kJ/mol
= 179.9 x 103 J/mol
T = 546K
Hence according to Arrhenius equation

= (- 5 + 0.3834) + 17.2081
= 12.5924 s-1
A = Antilog (12.5924)
A = 3.912 x 1012 s-1
Hence the value of pre-exponential factor is
3912 x 1012 s-1.
Question 27.
Consider a certain reaction A → Products with k= 20 x 10-2 s-2. Calculate the concentration of A remaining after 100 s if the initial concentration of A is 1.0 mol L-1.
Solution:
k = 20 x 10-2 s-1
[A]0 = 1.0 mol L-1
t = 100s
[A] = ?

– log [A] = 0.8684
log[A] = – 0.8684
[A] = Antilog (-0.8684)
or [A] = Antilog (1.1316)
[A] = 0.1354 mol L-1
Hence after 100s, the remaining amount of concentration will be 0.1354 mol L-1
Question 28.
Sucrose decomposes in acid solution into glucose and fructose according to the first order rate law with t1/2 = 3.00 hours. What fraction of the sample of sucrose remains after 8 hours?
Solution:
For a first order reaction

Let the initial concentratiorn is 1mol/L, then
[A]0 = 1
[A] = 
[A] = 0.158 mol/L
Hence after 8 hours 0.158 M sucrose will remain.
Question 29.
The decomposition of a hydrocarbon follows the equation k= (45 x 1011s-1)e-28000 k/T Calculate activation energy (Ea).
Solution:
According to Arrhenius equation,
k= Ae-Ea/RT …. (1)
According to equation
k= (4.5 x 1011 s-1)e-28000 k/T …..(2)
Compare equation (1) and (2)
or Ea = 28000 K x R
or Ea = 28000 K x 8.314J K-1mol-1
Ea = 232792 J/mol
Ea = 232.79 kJ/mol
The activation energyis 232.79 kJ/mol
Question 30.
The rate constant for the first order decompostion of H2O2 is given by the following eqaution:
log k = 14.34 – 125 x 104 KT
Calculate Ea for this reaction and at what temperature will its t1/2 be 256 minutes?
Solution:
According to Arrhenius equation,


T = 669K
Hence temperature = 669 K
Question 31.
The decomposition of A into products has value of kas 4.5 x 103 s-1 at10°C and energy of activation is 60 kJ mol-1. At what temperature would kbe 15 x 104 s-1?
Solution:
k1 = 4.5 x 103s-1
T1 = 10 + 273 = 283 K
k2 = 1.5 x 104 s-1
T2 = ?
Ea = 60 kJ/mol = 60 x 103 J/mol
According to Arrhenius equation,

or 0.0472 T2 = T1 – 283
or T1 – 0.0472 T2 = 283
or 0.9528 T2 = 283
T2 =  = 297 K
= 297 K
Hence temperature = 297 K
Question 32.
The time required for 10% completion of a first order reaction at 298 K is equal to that required for its 25% completion at 308 K. If the value of A is 4 x 1010 s-1, Calculate the value of k at 318 K and Ea.
Solution:



= 10.6021 – 12.5843= – 1.9822
k = Antilog (-1.9822) = Antilog (2.0178)
k = 1.042 x 10-2 s-1
Question 33.
The rate of a particular reaction quadruples on increasing temperature from 293 K to 313 K. For this reaction, calculate activation energy.
Solution:
According to question,

= 528545 J mol-1
Ea = 52.854 kJ mol-1.
RBSE Class 12 Chemistry Chapter 4 Long Answer Type Question
Question 1.
The decompostion of dimethyl ether leads to the formation of CH4, H2 and CO and the reaction rate is given by, Rate = k [PCH3OCH3]3/2. If the pressure is measured in bar and time in minutes, then what are the units of the rate and rate constant?
Answer:
CH2OCH3 → CH4 + H2 + CO

Question 2.
What is the effect of temperature on the rate constant of a reaction? How can this temperature effect on the rate constant be represented quantitatively?
Answer:
The rate constant of a reaction increases with increase of temperature and becomes nearly double for every 10° rise of temperature. The effect can be represented quantitatively by Arrhenius equation,
k = Ae-Ea/RT
Where,
A = Arrhenius constant
Ea = Activation energy
R = Gas constant
T = Temperature
k = Rate Constant
Question 3.
A reaction is of first order with respect to A and second order with respect to B.
(i) Write differential rate equation.
(ii) How is the rate affected when the concentration of B is trippled?
(iii) How is the rate affected when the concentration of both A and B is doubled.
Answer:
(i) Rate of reaction
(ii) Let[A] = a, [B] = b
If [B] is increases three times
[B] = 3b
then,
rate = k[A] [B]2
rate1 = k × a × b2 ….(1)
rate2 = k x a x (3b)2 …(2)
from eq (1) and (2)
(rate)2 = 9 x (rate)1
The rate becomes nine times.
(iii) (rate) = k[A] [B]2
(rate)1 = k x a x b2
(rate)2 = k x (2a) x (2b)2

(rate)2 = 8 (rate)1
The rate becomes eight times.
Question 4.
The experimental data for decomposition of N2O5 [2N2O5 → 4NO2 + O2] in gas phase at 318 K are given below:
| Time/s1O2 x[N2 O5 ]/molL-1 | 0 1.63 | 400 1.63 | 800 1.14 | 1200 0.93 | 1600 0.78 |
| Time/s102x[N2O5]/ molL-1 | 2000 0.64 | 2400 0.53 | 2800 0.43 | 3200 0.35 |
(i) Plot (N2O5) aganist t.
(ii) Find the half life period for the reaction.
(iii) Draw a graph between log (N2O5) and t.
(iv) What is the rate law ?
(v) Calculate the rate constant.
(vi) Calculate the half life period from k and compare it with answer (ii).
Answer:
| t(s) | 0 | 400 | 800 | 1200 | 1600 | 2000 | 2400 | 12600 | 3200 |
| [N2O5J(mol L-1) | 1.63 | 1.36 | 1.14 | 0.93 | 10.78 | 0.64 | 0.53 | 10.43 | 0.35 x l0-2 |
| log N2O5 | -1.79 | -1.87 | – 1.94 | -2.03 | 2.11 | -2.19 | -2.28 | 1-2.37 | -2.46 |
(i) Plot (N2O3) aganist t

(ii) Half life period (t1/2) for the given reaction is the time during which initial concentration of N2O5 changes from 1.63 x 10-2 M to half this value i.e. to 0.815 x 10-2 M and in the graph it has been shown to be 1440 s.
(iii) Graph between log [N2O5] and time t.

(iv) Since a straight line is observed when log [N2O5] is plotted against time, therefore the reaction is of first order.







0 Comments