Chapter 15 Statistics Ex 15.2

Rajasthan Board RBSE Class 9 Maths Solutions Chapter 15 Statistics Ex 15.2

Question 1.
The weight of 30 students (in kg) of Class X of a secondary school are as follows:
34, 34, 36, 37, 38, 33, 34, 35, 36, 37, 38, 33, 34, 35, 34, 33, 37, 35, 34, 36, 38, 36, 35, 34, 35, 37, 38, 34, 35, 35.
Prepare a frequency table for it.
Solution:

Question 2.
The weight (in kg) of 30 newly born babies in a village are as follows:

Solution:

Question 3.
Three coins were tossed 30 times simultaneously. Each time the number of heads occurring was noted down as follows:

Prepare a frequency distribution table for the these data.
Solution:

Question 4.
The blood groups of 30 students of Class X are recorded as follows:
A, B, O, O, A, B, O, A, O, B, A, O, B, A, O, O, A, AB, O, A, A, O, O, AB, B, A, O, B, A, B, O.
Represent these data in the form of a frequency distribution table. Which is the most common, and which is the rarest, blood group among these students?
Solution.
We can represent the above data as follows:

From the above table, the most common blood group is O and the rarest blood group is AB.

Question 5.
The marks obtained by 30 students of Class IX in an examination are as follows. Prepare a frequency table of 5 classes of class size 10.
19, 27, 40, 3, 33, 41, 18, 8, 20, 0, 23, 49, 16, 36, 14, 39, 6, 12, 29, 28, 22, 24, 37, 10, 23, 38, 35, 9, 49, 23
Solution.
Maximum marks = 49; Minimum marks = 0
Range = Maximum marks – Minimum marks = 49 – 0 = 49
Class interval (size) = 10
Number of intervals =  = 4.9 = 5

Question 6.
Prepare a frequency table by taking 5 as width of the class interval from the following data 13, 11, 8, 19, 0, 44, 27, 10, 8, 35, 13, 27, 30, 17, 43, 23, 19, 43, 17, 7
Solution.
Maximum value = 44; Class size = 5 (given); Minimum value = 0
∴Range = 44 – 0 = 44
∴No of intervals =  = 9

Question 7.
The value of π upto 50 decimal places is given below.
3.14159265358979323846264338327950288419716939937510
(i) Make a frequency distribution table of the digits from 0 to 9 after the decimal point.
(ii) What are the most and the least frequency occurring digits?
Sol. (i) The required frequency distribution table is shown below:

(ii) The most frequently occurred digits are 3 and 9 while 0 occurs least frequently.

Question 8.
The distance (in km) of 40 engineers from their residence to their place of work were found as follows:

Construct a grouped frequency distribution table with class size 5 for the data given above taking the first interval as 0 – 5 (5 not included). What main features do you observe from this tabular representation.
Sol. We have:
Maximum distance (in km) = 32; Minimum distance (in km) = 2
Range = Maximum value – Lowest value = 32 – 2 = 30
Class size = 5 (given)
∴ Number of intervals =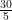  = 6

From the above table, we observe that 5 + 11 + 11 + 9 = 36 engineers live at a distance up to 20 km from their place of work. Only 4 engineers live at a distance of 20 km or more from their work place.

Question 9.
Thirty children were asked about the number of hours they studied in the previous week. The results were found as follows:

(i) Make a group frequency distribution table for these data, taking class width 5 and one of the class intervals as 5 – 10.
(ii) How many children studied for 15 or more hours in a week?
Solution.
(i) Maximum number of hours students studied = 17
Minimum number of hours students studied = 1
Range = Maximum value – Minimum value =17-1 = 16
Class width (size) = 5
Number of intervals =  = 3.2 i.e. 4

(ii) Number of children, who studied for 15 or more hours in a week are 2 in number.