Chapter 3 Multiple Choice Type Questions
Question 1.
Electric potential at any point due to a point charge is 300 volt and intensity of electric field is 50 V/m. The distance of charge of that point is.
(a) 9 m
(b) 15 m
(c) 6 m
(d) 3 m
Answer:
(c) 6 m
Electric field intensity E =

Question 2.
The charges are placed at corners of a square as shown in figure. Let the electric field and electric potential at its centre be

and V respectively. If the charges at A and B are displaced with respect to the charges at C and D, then :
(a) V changes and

remains unchanged
(b) Both

and V changes
(c) Both

and V remains unchanged
(d)

changes and V remains unchanged
Answer:
(d)

changes and V remains unchanged
Electric field

is changed while electric potential (V) remains unchanged
Question 3.
The potential at a point in an electric field is 200 V, then the work done in bringing an electron to that point is :
(a) -3.2 × 10-17J
(b) 200 J
(c) -200 J
(d) 100 J
Answer:
(a) -3.2 × 10-17J
Work done W = qv
W = -1.6 × 10-19 × 200
⇒ W = -3.2 × 10-17 J
Question 4.
Two charged conducting sphere of radii r1 and r2 are at same potential, then the ratio of the surface charge densities will be :
Answer:
(a)
Question 5.
A charge of 10 μC is situated at origin point on an X-Y coordinates. The potential difference between the points (α, 0) and

will be (in volts)
(a) 9 × 104
(b) Zero
(c)

(d)

Answer:
(b) Zero
Question 6.
The electric potential at the surface of a charged conducting hollow sphere of radius 2 m is 500 volt. The potential at the distance of 1.5 m from the centre is :
(a) 375 V
(b) 250 V
(c) zero
(d) 500V
Answer:
(d) 500V
Electric potential inside and on surface of charged conducting sphere will be same.
Electric potential inside the hollow spherical conductor is equal to the potential at the surface of the conductor.
∴ V = 500 Volt
Question 7.
The kinetic energy of a α-particle to move from the points where potential have 70 V to 50 V from rest is :
(a) 20 eV
(b) 40 eV
(c) 20 MeV
(d) 40 MeV
Answer:
(b) 40 eV
Work done W = qV
W = (2e)[VB – VA]
= (2e) [20] volt or
W = 40 eV
Question 8.
The electric field intensity is zero at the place, then the variation of potential with distance in that field will be :
(a) V ∝

(b) V ∝

(c) V = zero
(d) V = constant
Answer:
(d) V = constant
Electric field intensity
Question 9.
Two charged conducting spheres of equal surface charge densities have radii R
1 and R
2 If the potential at the centre is V
1 and V
2 respectively, then

is :
Answer:
(a)
Question 10.
The function of potential in an electric field is given by V = -5x + 3y +

. The electric field intensity at point (x, y, z) in S.I. system will be :
(a)

(b)

(c)

(d) 7
Answer:
(d) 7
Question 11.
A unit charge is rotated around a charge of q in a circular path at the distance of r. Then, work done will be :
(a) zero
(b)

(c) 2 πr J
(d) 2πrq J
Answer:
(a) zero
Circular path will act like a equipotential surface. So work done in moving unit charge will be zero.
Question 12.
The electric potential energy of a system in bringing an electron towards another electron is :
(a) increases
(b) decreases
(c) remains the same
(d) becomes zero
Answer:
(a) increases
On bringing similar charges to near the electric potential energy increases.
Question 13.
1000 small water drops, each of radius r and charge q, coalesce to form a single big drop. The potential of big drop, how much times the small drop is.:
(a) 1000
(b) 100
(c) 10
(d) 1
Answer:
(b) 100
Vlarge = n2/3Vsmall
Vlarge = (103)2/3Vsmall
[∵ n = 1000, n = 103]
∴ Vlarge = 102Vsmall = 100Vsmall
Question 14.
As shown in figure work done in bringing a coulomb charge from P to Q, due to the arranged charges will be :
(a) 10
(b) 5
(c) ∞
(d) zero
Answer:
(d) zero
WPQ = qV0 [VQ – VP]
WPQ = 1 [0 – 0]
WPQ = 0
Question 15.
64 identical mercury ball (each of potential 10 V) combine to form a big ball, then the potential at the surface of the big ball will be :
(a) 80 V
(b) 160 V
(c) 640 V
(d) 320 V
Answer:
(b) 160 V
Vlarge = n2/3Vsmall
Vlarge = (64)2/3 × 10
Vlarge = (43)2/3 × 10
Vlarge = 160 Volt
RBSE Class 12 Physics Chapter 3 Very Short Answer Type Questions
Question 1.
Is the electric potential a scalar or vector quantity?
Answer:
Scalar quantity.
Question 2.
Define electric potential.
Answer:
The electric potential at a point is equal to the work done by external force in bringing the unit positive charge from infinity to a point inside the electric field without changing the kinetic energy.
Question 3.
Can two equipotential surfaces intersect each other?
Answer:
No two equipotential surfaces or places can not intersect each other because then there would be two values of electric potential at the intersection point, which is impossible.
Question 4.
What would be the potential at infinity due to an isolated charge?
Answer:
V =

, r → ∞ ∴ V = 0
Question 5.
Can electric potential be zero at a point in a vacuum, the electric field at that point is not zero. Give example.
Answer:
Yes, it is possible.
Example :
- Between line joining electric dipole
- On equatorial line of an electric dipole.
Question 6.
Can electric field at a point be zero, but the electric potential is non-zero. Give example.
Answer:
Yes, it is possible.
Example :
- Inside uncharged spherical shell and charged conductor electric field is zero but electric potential cannot zero.
- Between the line joining two similar charges of equal magnitude.
Question 7.
How much work is done between the points at the distance 10 cm on an equipotential surface in taking the charge of 200 μC?
Answer:
W = q0[VB – VA]
On equipotential surface,
VB = VA ⇒ W = 0
Question 8.
What would be the shape of the equipotential surfaces due to :
(a) A point charge
(b) A uniform electric field
Answer:
(a) Equipotential surface due to point charge is spherical.
(b)
Question 9.
What is the electric potential energy when an electric dipole is placed parallel to the electric field?
Answer:
U = -pE cosθ
θ = 0°, cos(0) = 1
U = -pE
Question 10.
The potential on charging the surface of a conducting sphere of radius 10 cm is 15 V. What is the potential at its centre?
Answer:
On surface and inside surface the electric potential will be same. Therefore at centre the electric potential will be 15 volt.
Question 11.
The potential on a uniformly charged non-conducting sphere of radius 5 cm is 10 V. What is the potential at its centre?
Answer:
Question 12.
The electric potential at a point (x, y, z) (all in metres) in vacuum is V = 2x2 volt, Calculate electric field intensity at (1 m, 2 m, 3 m).
Answer:
Question 13.
Write the formula for potential energy for a system of two point charges.
Answer:
Question 14.
Write the formula for potential energy for a System of three point charges.
Answer:
Question 15.
Write the unit of potential gradient.
Answer:
Volt/metre.
Question 16.
How much work is done in taking an electron between two points that have potential difference of 20 V?
Answer:
W = q0V = 1.6 × 10-19 × 20 = 32 × 10-19 J
Question 17.
The electric potential at a point in vacuum due to another point charge is 10 V. If a material of dielectric constant 2 is placed
around the point, then what electric potential?
Answer:
Question 18.
What is the work done in rotating an electric dipole in an external uniform electric field

from 0° to 180°?
Answer:
W = pE(cosθ1 – cosθ2)
θ1 = 0°, θ2 = 180°
W = pE( cosθ°-cos180°)
= pE [1 – (1)]
⇒ W = 2pE Joule
Question 19.
What is the electric potential of earth?
Answer:
Zero.
Question 20.
If potential function, V = (4x+ 3y) V, then what is the electric field intensity at point (2, 1) (all in metres)?
Answer:
Chapter 3 Short Answer Type Questions
Question 1.
What is electric potential? Write its formula and unit.
Answer:
Electric Potential
In fig. 3.1 it is shown that in electric field due to a configuration of charges, the work done in carrying a test charge (q0) from point A to B, depends only upon the distance between A and B not on the path adopted.
“Electric potential is the factor which decides the direction of flow of electric charge.”
It is scalar quantity and it is denoted by V. As liquids flow from higher gravitation level to the lower level; heat flows from higher temperature to the lower temperature, similarly charge (positive) flows from higher electric potential to the lower potential. Negative charge flows from lower electric potential to the higher potential.
If VA and VB be the electric potentials at points A and B respectively, then the potential difference between points A and B is given below,
V
B – V
A =

……………… (1)
Where, WAB is the work done in carrying test charge + q0 from A to B.
Here we assume that in this process, the test charge does not disturb the source of field, i.e., all the charges remain at their position. If for a displacement, the change in potential energy is UB — UA, then the potential difference between the points A and B can be given as
If q0 = +1C, then VB – VA = WAB
Question 2
Prove that the potential inside a charged spherical shell is equal to that on its surface.
Answer:
Inside the charged spherical shell (r < R)
According to definition of potential
Dependence of electric field upon distance is different e.g. outside spherical shell
E =

and inside the shell E = 0.
Therefore the integration can be written as under,
In other words, E = 0
By the relation of electric field and potential
Question 3.
What is equipotential surface? Draw the equipotential surface due to point charge.
Answer:
Equipotential Surface
The surface in an electric field where the value of electric potential is same at all the points on the surface is called equipotential surface. The potential difference between two points in an equipotential surface is zero. Thus, work done in moving the charge from one point to another in an equipotential surface is zero. Since the work done is zero when the direction of force (electric field) and displacement is normal. Thus, electric field is perpendicular to the plane in an equipotential surface.
It is to be noted for equipotential surface :
1. For a uniform electric field

, the equipotential surface is normal to its field lines. According to figure 3.8, I (a) II and III are equipotential surfaces.
2. For an isolated point charge :
Potential at a distance r due to point charge +q,
If we draw a sphere of radius r surrounding the +q charge. Then, potential will be same at all points on the sphere i.e., equipotential surfaces are spherical whose charge is at the centre. For a positive point charge, as the radius of the spherical surface increases, the potential of spherical surface decreases.
3. Equipotential surface for an electric dipole : The equipotential surface for an electric dipole are shown dotted in figure 3.8 (c).
4. Equipotential surfaces for a pair of similar point charges : The equipotential surface is as shown in figure 3.8 (d)
Question 4.
Determine the electric potential energy for a system of three point charges.
Answer:
Electric Potential Energy of a System of Charges
Electric potential energy of a system of charges is equal to amount of work done in forming the system of charges by bringing them at their particular positions from infinity without any acceleration and against the electrostatic force. It is denoted by U.
U = W = qV(r)
(a) Electric potential energy of system of two charges :
Suppose two charges + q1 and + q2 are situated at a distance r. We have to find out the electrical potential energy of this system.
When the charge q1 is brought from infinity to in its position, no work is done because there is no other charge to repel or attract it. The electric potential due to this charge q1 at a distance r i.e., in the position of charge q2,
V
1 =

Therefore the work done in bringing the charge q2 from infinity to its own position i.e., the electric potential energy of the system.
W = U = V1q2
or U =

……………. (1)
If both the charges are of same nature, the potential energy will be positive and for unlike charges it will be negative. Therefore during the process of evaluation of potential energy, the value of charge should be used with its sign.
(b) Electric potential energy of a system of three charges :
Let us first of all consider a system of three point charges q1, q2 and q3. Such that q2 and q3 are initially at infinite distance from the charge (q1) [In figure 3.18(a)], Work done to bringing the charge q2 from infinity to point B,
W
12 =

…………… (2)
Work done bringing charge q3 (In the presence of charge q1 and q2) from infinity to point C
Now the total work done to bringing all the three charges from infinity to their respective position is given by
This work done is stored in the form of potential energy
∴ U = W = potential energy of three system of
(c) Electric potential energy due to four system of charges :
Suppose there are four charges in a system of charges, situated as shown in figure (3.18 (b)).
The total energy of this system can be obtained by adding the potential energies of each possible pair.
Similarly, the electrical potential energy of the system of n charges can be obtained. We can write the equivalent expression as under,
To obtain this sum, we have to use each pair of charge only once. Therefore the general formula for electrical potential energy will be as under
Here we have to multiply the expression by
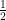
because in this expression each pair comes two times.
Question 5.
Why is static electric potential of the whole volume of the charged conductor equivalent to that on its surface?
Answer:
Electric field inside whole volume of charged conductor is zero.
∵ E =

E = 0 ⇒ V = constant
(Electric potential is uniform in whole volume)
Inside conductor the work done in bringing any test charge from inside point to surface will be zero.
W = q0(VB – VA)
VB – VA = 0
∴ VA = VB
Potential of both points will be equal.
Question 6.
Establish the relation between electric potential and electric field intensity.
Answer:
Relation between Electric Field and Electric Potential
We have seen the relation between electric field and electric potential. With the help of this relation, we can evaluate potential difference between two points in known electric field.
In this section, our aim is to determine electric field for known potential function V.
For an electric field

, the small element

of displacement can be written in the form of derivative.
dV =

= -Edl cosθ ………….. (1)
Where θ is the angle between electric field (

) and length (

)

= Ecosθ
The term

represents decrease in potential with distance. It is clear from the above equation, that if the angle between

and

, θ = 0°, then the loss in potential with distance is maximum. In general form, the term is a scalar quantity but its maximum value

in a particular direction (θ = 0) i. e.. in the direction of electric field. Thus, the maximum rate of loss in potential can be considered to be a vector which is along

. In mathematical language, it is called potential gradient and it is represented as Grad V.
For an equipotential surface, the direction of Grad V is perpendicular to the surface. It can be understood with the help of figure 3.9. The equipotential surfaces are shown as S
1and S
2 on which the potential is V and V-dV respectively. The loss in potential in moving from point A on surface S
1 to points B and C is dV, but the rate of change in potential with distance is

and

respectivelv,
which are different from each other.
Since, AB < AC
Thus,

Now, surface AB is perpendicular to the surface. Thus, the rate of loss in potential is maximum in the direction normal to the surface.
Equation (1) can be written as :
where, E
1 = Ecos
1, component of

along

. Please note that we have used the symbols of partial derivatives which tells that the above equation in a definite axis (here for l axis) sums up the change in potential and component of E along that axis. If we assume l axis to be X, Y and Z axes, then the components of

will be
This operator is called Del operator. With the help of equation (5), we can find out

when the potential function V(x, y, z) is known.
If the potential function is a function of radius r. Then radial electric field
E
r =

……………… (7)
Question 7.
Obtain the formula for rotating the electric dipole in a uniform electric field.
Answer.
Work Done in Rotating of an Electric Dipole In a Uniform Electric Field
On placing an electric dipole in an uniform electric field, a torque is produced on the dipole which tries to align it in the direction of the electric field. Ultimately the dipole gets aligned with the field and attains equilibrium. Thus it is obvious that work will be done in rotating the dipole in uniform electric field.
The torque acting on an electric dipole when it is placed in a uniform electric field

making an angle θ with the field,
τ = pEsinθ ………(1)
Where p(= q × 2l) is electric dipole moment and E, the intensity of electric field.
Therefore work, done in giving an angular displacement dθ,
dW = τdθ
If the dipole is rotated from θ1 orientation to θ2,
Question 8.
Show that no work is done in taking a test charge from one point to another in an equipotential surface.
Answer.
The potential difference between any two points on an equipotential surface ΔV = 0,
Work done = q0V = 0
Question 9.
What is meant by electric potential energy? Obtain the formula for potential energy for a system of charges.
Answer:
Electric Potential Energy of a System of Charges
Electric potential energy of a system of charges is equal to amount of work done in forming the system of charges by bringing them at their particular positions from infinity without any acceleration and against the electrostatic force. It is denoted by U.
U = W = qV(r)
(a) Electric potential energy of system of two charges :
Suppose two charges + q1 and + q2 are situated at a distance r. We have to find out the electrical potential energy of this system.
When the charge q1 is brought from infinity to in its position, no work is done because there is no other charge to repel or attract it. The electric potential due to this charge q1 at a distance r i.e., in the position of charge q2,
V
1 =

Therefore the work done in bringing the charge q2 from infinity to its own position i.e., the electric potential energy of the system.
W = U = V1q2
or U =

……………. (1)
If both the charges are of same nature, the potential energy will be positive and for unlike charges it will be negative. Therefore during the process of evaluation of potential energy, the value of charge should be used with its sign.
(b) Electric potential energy of a system of three charges :
Let us first of all consider a system of three point charges q1, q2 and q3. Such that q2 and q3 are initially at infinite distance from the charge (q1) [In figure 3.18(a)], Work done to bringing the charge q2 from infinity to point B,
W
12 =

…………… (2)
Work done bringing charge q3 (In the presence of charge q1 and q2) from infinity to point C
Now the total work done to bringing all the three charges from infinity to their respective position is given by
This work done is stored in the form of potential energy
∴ U = W = potential energy of three system of
(c) Electric potential energy due to four system of charges :
Suppose there are four charges in a system of charges, situated as shown in figure (3.18 (b)).
The total energy of this system can be obtained by adding the potential energies of each possible pair.
Similarly, the electrical potential energy of the system of n charges can be obtained. We can write the equivalent expression as under,
To obtain this sum, we have to use each pair of charge only once. Therefore the general formula for electrical potential energy will be as under
Here we have to multiply the expression by
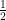
because in this expression each pair comes two times.
Question 10.
Obtain the formula for electric potential energy of an electric dipole in an external electric field.
Answer:
Electric Potential Energy of an Electric Dipole in an External Electric Field
Electric potential energy of an electric dipole in an electric field is equal to work done in bringing an electric dipole from infinity to that field.
In figure 3.21, an electric dipole is brought from infinity to a uniform electric field

so that the dipole moment

is always along electric field

. The force on charge +q due to electric field

,

= q

along the field and force on charge -q,

= -q

is opposite to the field. Thus, an external work is done for bringing charge q of dipole in electric field where as the electric field itself Work on charge -q. On coming from infinity to electric field, -q charge has to move 2a more distance that by q. Thus, the work done by the charge -q is more and negative. Thus, work done by the electric field,
W = Force on charge (-q) × Distance covered
W = -qE × 2a = -2 qaE
W = -pE ∵ p = 2qa
Thus, potential energy of electric dipole placed parallel to electric field

.
U1 = -pE ……………. (1)
Now, work done in rotating by angle θ from the parallel position of electric field

.
U2 = pE (1 – cosθ) ………….. (2)
Thus, potential energy of electric dipole at angle θ in electric field,
U = U1 + U2
U = -pE + pE (1 – cosθ)
U = -pE cosθ
In vector form, U =

…………. (3)
Equation (2) is the expression for potential energy of electric dipole.
Special Cases
(a) If the electric dipole is at 0° angle from the electric field, then the potential energy
U = -pE cosθ
U = -pE cos0°
i.e., U = -pE …………. (4)
(It is a condition of stable equilibrium)
(b) If electric dipole moment is perpendicular to the electric field, then the potential energy
U = -pEcos 90°
U = 0 ………….. (5)
(c) If the electric dipole moment is at 180° angle from the electric field, then the potential energy,
U = -pE cos 180°
U = pE …………….. (6)
(It is a condition of unstable equilibrium)
Case (a) is called the state of stable equilibrium becuase in this case, energy is minimum and case (c) is called the unstable equilibrium because in this case, the energy is maximum.
Question 11.
Write the formula for electric potential energy for two point charges q
1 and q
2 placed at displacement

and

respectively in a uniform external electric field.
Answer:
Electric Potential Energy of Charges in an External Electric Field
(i) Electric potential energy of a single charge in an external field : Let us consider an external electric field (

) have different values of electric potential at different points. Consider a point ‘ P’ at the distance (r) from the origin in this field having a electric potential

. Then, work done to bringing a charge (q) from infinite to point ‘ P’ is given by q

. This work is used as a potential energy of charge (q). Thus the potential energy of charge in external field.
(ii) Potential energy of a system of two charges in an external field : Let q
1 and q
2 be two charges placed at points P and Q having position vectors

and

respectively. In bringing (q
1) from infinity to point P, work done =

, where

is the potential at P due to external electric field.
To bringing (q
2) from infinity to point Q, work done =

where

is the potential at Q due to external electric field. If

is the distance between point P and point Q, then work done on q
2 against the
field due to q
1 =

∴ The total potential energy of two charges
Question 12.
Write any two properties of equipotential surface.
Answer:
- No two equipotential surfaces or planes cut each other because then there would be two values of electric potential at the intersection point, which is not possible.
- The surface of a conductor is always equipotential. Actually, the whole volume of a conductor is at equal potential.
Equipotential surfaces indicate regions of strong or weak electric fields. Using E =

or dr =

since dV (i.er potential difference) is constant on the equipotential surface, so dr ∝

- If E is strong, dr will be small and the separation of equipotential surfaces will small than the electric field is strong. The separation between equipotential surface is large than the electric field is weak.
Question 13.
Prove that the electric potential of point charge which has a dielectric medium around it, is 1/εr times lesser than that in vacuum.
Answer:
Electric potential at r distance from charge +q is
V =

If a dielectric medium of er is surrounded by charge, then electric potential.
Question 14.
Prove that the electric potential at the centre of a uniformly charged non-conducting sphere is 1.5 times more than that on the surface.
Answer:
Electric Potential due to Charged Non-conducting Sphere
Consider a non-conducting sphere of radius R be charged by a charge q. The electric field intensity at the points outside the sphere, on the surface and inside the sphere is as follows :
The potential at a point in electric field intensity

is calculated by the help of following relation
The electric potential is calculated at the observation point in different conditions :
(a) At a point outside the non-conducting sphere
(b) At a point on the surface of non-conducting sphere
Putting r = R in equation (1),
V
S =

……………. (2)
(c) At a point inside the non-conducting sphere (r < R)
For a point inside the non-conducting sphere, the electric field intensity E does not depend uniformly from infinity to r distance, i.e., from infinity to surface, it is inversely proportional to the square of the distance r and it is directly proportional to the distance from the surface to the centre r. Thus, integral is divided into two parts :
Thus, the electric potential at centre of a charged non-conducting sphere is 1.5 times that on its surface.
It is clear that the electric potential decreases with r2 from centre to surface in a charged non-conducting sphere. After that, it decreases as per the law of r-1 and becomes zero at infinity. It is shown in a graph in figure (3.16),
Question 15.
Two charges 10μC and 5μC are at lm apart. In order to make this distance 0.5 m, how much work would be done?
Answer:
q1 = 10μC = 10 × 10-6 C
q2 = 5μC = 5 × 10-6 C
Question 16.
Define electric potential difference. Distinguish between electric potential difference and electric potential.
Answer:
Electric Potential and Potential Difference
In fig. 3.1 it is shown that in electric field due to a configuration of charges, the work done in carrying a test charge (q0) from point A to B, depends only upon the distance between A and B not on the path adopted.
“Electric potential is the factor which decides the direction of flow of electric charge.”
It is scalar quantity and it is denoted by V. As liquids flow from higher gravitation level to the lower level; heat flows from higher temperature to the lower temperature, similarly charge (positive) flows from higher electric potential to the lower potential. Negative charge flows from lower electric potential to the higher potential.
If VA and VB be the electric potentials at points A and B respectively, then the potential difference between points A and B is given below,
V
B – V
A =

……………… (1)
Where, WAB is the work done in carrying test charge + q0 from A to B.
Here we assume that in this process, the test charge does not disturb the source of field, i.e., all the charges remain at their position. If for a displacement, the change in potential energy is UB – UA, then the potential difference between the points A and B can be given as
If q0 = +1C, then VB – VA = WAB
Chapter 3 Long Answer Type Questions
Question 1.
Deduce an expression for electric potential due to a charge at any point?
Answer:
Electric Potential due to a Point Charge
According to definition electric potential at a point is equal to the amount of work done in bringing unit positive test charge from infinity to that point in the electric field without any acceleration.
Suppose a point charge +q is placed at point O and potential due to this charge at point P situated at distance r from the charge, is to be determined. According to definition of potential, to find out the potential at P, we have to estimate the work done in bringing +1C charge from infinity to point P inside the electric field. For this purpose, let us consider another point P’ situated at distance x from O beyond P and + q0 positive test charge is placed at P’, the electrostatic force acting on +q0,
F =

Work done in giving a small displacement dx toward P,
dW =

= Fdxcos 180° = Fdx(-1)
or dW = -F dx
Therefore work done in bring +q0 charge from infinity to P
Where V → potential at P
If q is positive then potential V will also be positive and if q is negative, then potential will also be negative.”
From equation (1)
V ∝

Electric field due to point charge
E =

∴ E ∝

If V and E both are plotted with distance r then the graphs will be as under figure [3.4(a)].
For negative charge V — r graph will be as under figure [3.4(b)].
Question 2.
Derive an expression for electric potential due to an electric dipole at any point (r, 0). Prove that the electric potential at point on axial is maximum and at equatorial is zero?
Answer:
Electric Potential due to an Electric Dipole at Any Point
An electric dipole AB is shown in figure 3.7. The charges at points A and B are -q and +q respectively and the distance between them is 2a. We have to determine the electric potential at point P from its centre O at distance r and the line OP makes an angle 0 with the axis of the dipole.
Now, to calculate (r1 – r2) and r1r2, we draw perpendiculars on AC and BD from points A and B on line OP respectively.
It is clear that potential due to dipole depends on r-2 and not on r-1 (as due to single charge).
Special Cases :
(i) If point P is on the axis when θ = 0°, cosθ = 1, then from equation (4),
V =

(ii) If the point is normal, then θ = 90° , cosθ = 0, then from equation (4),
V = 0
In vector form, potential at point (r, θ) due to dipole,
Question 3.
Derive an expression for potential due to charged spherical shell at following points (i) outer, (ii) at surface, (iii) inner point and also draw the graph for the variation of potential with the distance.
Answer:
Electric Potential due to Charged Spherical Shell
(a) At point P outside the shell (r > R)
When unit positive test charge is brought from infinity to point P, then the potential at P is equal to negative value of line integral of electric field between infinity and P.
Therefore, according to definition of potential
(b) On the surface of the charged spherical shell (r = R)
According to the definition of potential,
Where V is constant
(c) Inside the charged spherical shell (r < R)
According to the definition of potential
Dependence of electric field upon distance is different e.g. outside spherical shell
E =

and inside the shell E = 0.
Therefore the integration can be written as under,
Question 4.
Find the expression for electric potential due to an insulated charged sphere at outer point, surface and inner point?
Answer:
Electric Potential due to Charged Non-conducting Sphere
Consider a non-conducting sphere of radius R be charged by a charge q. The electric field intensity at the points outside the sphere, on the surface and inside the sphere is as follows :
The potential at a point in electric field intensity

is calculated by the help of following relation
The electric potential is calculated at the observation point in different conditions :
(a) At a point outside the non-conducting sphere
(b) At a point on the surface of non-conducting sphere
Putting r = R in equation (1),
V
S =

……………. (2)
(c) At a point inside the non-conducting sphere (r < R)
For a point inside the non-conducting sphere, the electric field intensity E does not depend uniformly from infinity to r distance, i.e., from infinity to surface, it is inversely proportional to the square of the distance r and it is directly proportional to the distance from the surface to the centre r. Thus, integral is divided into two parts :
Thus, the electric potential at centre of a charged non-conducting sphere is 1.5 times that on its surface.
It is clear that the electric potential decreases with r2 from centre to surface in a charged non-conducting sphere. After that, it decreases as per the law of r-1 and becomes zero at infinity. It is shown in a graph in figure (3.16),
Question 5.
Give the definition of electric potential energy. Find out the expression for electric potential energy of a dipole in uniform electric field. In which condition get the position of stable or unstable equilibrium
Answer:
Electric Potential Energy of an Electric Dipole in an External Electric Field
Electric potential energy of an electric dipole in an electric field is equal to work done in bringing an electric dipole from infinity to that field.
In figure 3.21, an electric dipole is brought from infinity to a uniform electric field

so that the dipole moment

is always along electric field

. The force on charge +q due to electric field

,

= q

along the field and force on charge -q,

= -q

is opposite to the field. Thus, an external work is done for bringing charge q of dipole in electric field where as the electric field itself Work on charge -q. On coming from infinity to electric field, -q charge has to move 2a more distance that by q. Thus, the work done by the charge -q is more and negative. Thus, work done by the electric field,
W = Force on charge (-q) × Distance covered
W = -qE × 2a = -2 qaE
W = -pE ∵ p = 2qa
Thus, potential energy of electric dipole placed parallel to electric field

.
U1 = -pE ……………. (1)
Now, work done in rotating by angle θ from the parallel position of electric field

.
U2 = pE (1 – cosθ) ………….. (2)
Thus, potential energy of electric dipole at angle θ in electric field,
U = U1 + U2
U = -pE + pE (1 – cosθ)
U = -pE cosθ
In vector form, U =

…………. (3)
Equation (2) is the expression for potential energy of electric dipole.
Special Cases
(a) If the electric dipole is at 0° angle from the electric field, then the potential energy
U = -pE cosθ
U = -pE cos0°
i.e., U = -pE …………. (4)
(It is a condition of stable equilibrium)
(b) If electric dipole moment is perpendicular to the electric field, then the potential energy
U = -pEcos 90°
U = 0 ………….. (5)
(c) If the electric dipole moment is at 180° angle from the electric field, then the potential energy,
U = -pE cos 180°
U = pE …………….. (6)
(It is a condition of unstable equilibrium)
Case (a) is called the state of stable equilibrium becuase in this case, energy is minimum and case (c) is called the unstable equilibrium because in this case, the energy is maximum.
Chapter 3 Numerical Questions
Question 1.
6 J of work is done to take a charge of 3 C between two points. Calculate the electric potential difference between these points.
Solution:
Potential difference between A and B
Question 2.
If the electric potential between two points A and B is 2 V and 4 V respectively. Then, how much work is done in bringing a point charge of 8 μC from point A to point B?
Solution:
q0 = 8μC
q0 = 8 × 10-6C
Potential differnce between A and B
V
B – V
A =

4 – 2 =

WAB = 2 × 8 × 10-6
WAB = 16 × 10-6 J
WAB = 1.6 × 10-5 J
Question 3.
Four charges 100 μC, -50 μC, 20 μC and -60 μC are placed at the corners of a square of side
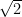
m. Calculate the electric potential at the centre of the square.
Solution:
According to figure
Diagonal of square
Question 4.
Two charges 3 × 10-8C and -2 × 10-8C are 15 cm apart. At what point on the line joining the charges, the electric potential is zero? Let the electric potential at infinity is zero.
Solution:
Potential at point P
V1 + V2 = 0
x = 0.45m = 45 cm
Question 5.
Each side of a square is 0.9 m long. The charges at its corners are -2 μC, + 3 μC, – 4 μC and +5μC. Determine the electric potential at the centre of the square.
Solution:
Question 6.
Charge of 5 μC is at each of the vertices of a regular hexagon of side 10 cm. Determine the electric potential at the centre of the regular hexagon.
Solution:
For Hexagonal shape
OA = OB – OC = OD = OE = OF = 10cm = 0.1 m
Potential at O
Question 7.
Charge of 2μC is placed at each corner of the square ABCD of side

cm. Calculate the electric potential at the centre of the square.
Solution:
Question 8.
The side of an equilateral triangle is 100 cm. The charges at its three corners are 1 μC, 2 μC and 3 μC respectively. Calculate the electric potential at a point (in the centre) equidistant from the three corners of the triangle.
Solution:
Given a = 100 cm
= 100 × 10-2 m
Draw a ⊥OD and AB in ∆ ODB
Question 9.
The distance between the charges -1 μC and +1 μC of an electric dipole is 4 × 10-14 m. Calculate the potential at an axial point 2 × 10-6m from the centre of the dipole.
Solution:
Question 10.
(a) Calculate potential at a point at 9 cm distance due to charge 4 × 10-7 C.
(b) Determine the work done in bringing a charge of 2 × 10-9 C from infinity to that point. Does this work depend upon the path along which it was brought?
Solution:
(a) Potential at point P
(b) Work done in bringing charge 2 × 10-9 C from infinity to point P
W = qV
= 2 10-9 C × 4 × 104 V
= 8 × 105 J
This work done does not depend on path follows.
Question 11.
A charge of 30μC is at the reference point in the X-Y coordinate system. Calculate the potential difference between the points

and (a, 0).
Solution:
V
B – V
A =

VB – VA = 0
Potential difference = 0
Question 12.
Three point charges -q, + q and +q are placed at the points (0, α), (0, 0) and (0, α) respectively in the x-y plane. Prove that the potential V at the distance r on the line making an angle 9 with the axis would be
Solution:
Here (0, α) and (0,- α) are position of charges – q and +q. They form an electric dipole. Electric potential is V2 at point P due to this electric dipole.
Question 13.
How much work will be done in placing the charges +q, +2q, and +4q at the corners of the equilateral triangle of side α metre?
Solution:
Question 14.
Two charges 7 μC and -2μC are placed at (-9 cm, 0, 0) and (+9 cm, 0, 0) respectively. There is no external field on the system. Determine the electrostatic potential energy of this system.
(b) How much work will be done in keeping the two charges at infinite distance from each other?
Solution:
(a) q1 = 7μC
q1 = 7 × 10-6 C
q2 = -2pC
q2 = -2 × 10-6C
r = 18cm
r = 18 × 10-2 m
(b) Potential energy at infinity
U2 = 0
Potential energy at given position
U1 = -0.7J
Work done in seperating the charges upto infinite
W = U2 – U1
⇒ W = 0 – (-0.7)
W = 0.7 J
Question 15.
The electric potential at a point (x, y) in an electric field is given by V = 6xy + y2 – x2 .
Calculate the electric field at that point.
Solution:
Question 16.
A charge of +15 μC is given to a hollow metallic sphere of radius 0.2 m. Determine;
(i) electric potential on the spherical surface
(ii) electric potential at the centre of the sphere
(iii) electric potential at 0.1 m from the centre of the sphere
(iv) electric potential at 0.3 m from the centre of the sphere.
Solution:
R = 0.2 m
V = 45 × 104
V = 4.5 × 105 volt
Question 17.
Three point charges +q, +2q and xq are placed at the corners of an equilateral triangle of side of length r. Calculate the value of x at which the potential energy of the system is zero?
Solution:
U = U12 + U13 + U3



















































or dr =
since dV (i.er potential difference) is constant on the equipotential surface, so dr ∝

























































0 Comments