Rajasthan Board RBSE Class 10 Maths Chapter 9 Co-ordinate Geometry Ex 9.2
Question 1.
Find the co-ordinates of the point which divides the line segment joining the points (3, 5) and (7, 9) in the ratio 2 : 3 internally.
Solution :
Let point P(x, y) divides the line segment joining the point A(3, 5) and B(7, 9) internally in the ratio 2 : 3.

Hence, the coordinate of P is .
.
Find the co-ordinates of the point which divides the line segment joining the points (3, 5) and (7, 9) in the ratio 2 : 3 internally.
Solution :
Let point P(x, y) divides the line segment joining the point A(3, 5) and B(7, 9) internally in the ratio 2 : 3.

Hence, the coordinate of P is
Question 2.
Find the co-ordinates of the point which divides the line segment joining the points (5, -2) and in the ratio 7 : 9 externally.
in the ratio 7 : 9 externally.
Solution :
Let point P(x, y) divides the line segment joining the points A(5, -2) and B externaly in the ratio 7 : 9.
externaly in the ratio 7 : 9.
x1 = 5 y1 = -2 m1 = 7
x2 = -1 y2 = 4 m2 = 9
y2 = 4 m2 = 9
By formula of external division

Hence, the coordinate of P is
Find the co-ordinates of the point which divides the line segment joining the points (5, -2) and
Solution :
Let point P(x, y) divides the line segment joining the points A(5, -2) and B
x1 = 5 y1 = -2 m1 = 7
x2 = -1
By formula of external division

Hence, the coordinate of P is
Question 3.
Prove that origin O divides the line joining the points 4(1, -3) and B(-3, 9) in the ratio 1 : 3 internally. Find the co-ordinates of the points which externally divides the line.
Solution :
Let the point P(x, y) divides the line segment joining the point A(1, -3) and B(-3, 9) internally in the ratio 1 : 3.


Hence co-ordinate of P(x, y) is (0, 0). So origin O is divides the line segment joining the given points internally in the ratio 1 : 3.
Again point P(x, y) divides externally, then by formula of external division

Hence, co-ordinate of point P which divides externally = (3, -9).
Prove that origin O divides the line joining the points 4(1, -3) and B(-3, 9) in the ratio 1 : 3 internally. Find the co-ordinates of the points which externally divides the line.
Solution :
Let the point P(x, y) divides the line segment joining the point A(1, -3) and B(-3, 9) internally in the ratio 1 : 3.


Hence co-ordinate of P(x, y) is (0, 0). So origin O is divides the line segment joining the given points internally in the ratio 1 : 3.
Again point P(x, y) divides externally, then by formula of external division

Hence, co-ordinate of point P which divides externally = (3, -9).
Question 4.
Find the mid point of line joining the points (22, 20) and (0, 16).
Solution :
Let co-ordinates of mid point is P (x, y) which ¡s line joining the points A(22, 20) and B(0, 16).
x1 = 22, y1 = 20, x2 = 0, y2 = 16
By formula of mid point

Hence, co-ordinate of mid point P(x, y) = (11, 18)
Find the mid point of line joining the points (22, 20) and (0, 16).
Solution :
Let co-ordinates of mid point is P (x, y) which ¡s line joining the points A(22, 20) and B(0, 16).
x1 = 22, y1 = 20, x2 = 0, y2 = 16
By formula of mid point

Hence, co-ordinate of mid point P(x, y) = (11, 18)
Question 5.
In which ratio, x-axis divides the line segment which joins points (5, 3) and (-3, -2)?
Solution :
On x-axis, the ordinate of each point is zero. So let point P(x, 0) divides the line segment joining the points A(5, 3) and B(-3, -2) internally in the ratio m1 : m2

Hence, line segment joining the given points by x-axis divides internally in the ratio 3 : 2.
In which ratio, x-axis divides the line segment which joins points (5, 3) and (-3, -2)?
Solution :
On x-axis, the ordinate of each point is zero. So let point P(x, 0) divides the line segment joining the points A(5, 3) and B(-3, -2) internally in the ratio m1 : m2

Hence, line segment joining the given points by x-axis divides internally in the ratio 3 : 2.
Question 6.
In which ratio, y-axis, divides the line segment which joins points (2, -3) and (5, 6)?
Solution :
On y-axis, coordinate of point x is zero, so let point P(0, y) divides the line segment joining the point A(2, -3) and B(5, 6) externally in the ratio m1 : m2.
Then by formula of external division

⇒ 5m1 – 2m2 = 0
⇒ 5m1 = 2m2
⇒
⇒ m1 : m2 = 2 : 5
Hence, line segment joining these points divides externally in the ratio 2 : 5 by y-axis.
In which ratio, y-axis, divides the line segment which joins points (2, -3) and (5, 6)?
Solution :
On y-axis, coordinate of point x is zero, so let point P(0, y) divides the line segment joining the point A(2, -3) and B(5, 6) externally in the ratio m1 : m2.
Then by formula of external division

⇒ 5m1 – 2m2 = 0
⇒ 5m1 = 2m2
⇒
⇒ m1 : m2 = 2 : 5
Hence, line segment joining these points divides externally in the ratio 2 : 5 by y-axis.
Question 7.
In which ratio, point (11, 15) divides the line segment which joins (15, 5) and (9, 20)?
Solution :
Let the point P(1 1, 15) divides the line segment joining the point A(15, 5) and B(9, 20) internally in the ratio λ : 1
Hence x1 = 15 y1 = 5 m1 = λ
x2 = 9 y2 = 20 m2 = 1

⇒ 11(λ + 1) = 9λ + 15
⇒ 11λ + 11 = 9λ + 15
⇒ 11λ – 9λ = 15 – 11
⇒ 2λ = 4
λ = =
= 
∴ λ : 1 = 2 : 1
Hence, required ratio is 2 : 1.
In which ratio, point (11, 15) divides the line segment which joins (15, 5) and (9, 20)?
Solution :
Let the point P(1 1, 15) divides the line segment joining the point A(15, 5) and B(9, 20) internally in the ratio λ : 1
Hence x1 = 15 y1 = 5 m1 = λ
x2 = 9 y2 = 20 m2 = 1

⇒ 11(λ + 1) = 9λ + 15
⇒ 11λ + 11 = 9λ + 15
⇒ 11λ – 9λ = 15 – 11
⇒ 2λ = 4
λ =
∴ λ : 1 = 2 : 1
Hence, required ratio is 2 : 1.
Question 8.
If point P (3, 5) divides line segment which joins A(-2, 3) and B(x, y) in the ratio 4 : 7 internally, then find the co-ordinates of B.
Solution :
Let the coordinate of point B is (x, y)

Let the point P(3, 5) divides the line segment joining the points A(-2, 3) and B(x, y) internally in the inthe ratio4 : 7.
So, x1 = -2, y1 = 3 m1 = 4
x2 = x y2 = y m2 = 7
By section formula


Hence, the co-ordinate of point B is
If point P (3, 5) divides line segment which joins A(-2, 3) and B(x, y) in the ratio 4 : 7 internally, then find the co-ordinates of B.
Solution :
Let the coordinate of point B is (x, y)

Let the point P(3, 5) divides the line segment joining the points A(-2, 3) and B(x, y) internally in the inthe ratio4 : 7.
So, x1 = -2, y1 = 3 m1 = 4
x2 = x y2 = y m2 = 7
By section formula


Hence, the co-ordinate of point B is
Question 9.
Find the co-ordinates of point which trisects the line joining point (11, 9) and (1, 2).
Solution :
Let P(x1 y1) and Q(x2, y2) are required points which is trisect the line segment joining the points A(11, 9) and B(1, 2) respectively.
Let AP = PQ = BQ = x
PB = x + x = 2x
AQ = x + x = 2x

Hence point P divides line segment AB in the ratio 1 : 2 and point Q, divides the line segment Ab in the ratio 2 : 1.
By the section formula for point P,
m1 = 1, m2 = 2

∴ Hence the co-ordinate of point P is
for point Q,
m1 = 2, m2 = 1

So, the co-ordinate of Q =
Hence, required coordinate of point P and Q is and
and  respectively.
respectively.
Find the co-ordinates of point which trisects the line joining point (11, 9) and (1, 2).
Solution :
Let P(x1 y1) and Q(x2, y2) are required points which is trisect the line segment joining the points A(11, 9) and B(1, 2) respectively.
Let AP = PQ = BQ = x
PB = x + x = 2x
AQ = x + x = 2x

Hence point P divides line segment AB in the ratio 1 : 2 and point Q, divides the line segment Ab in the ratio 2 : 1.
By the section formula for point P,
m1 = 1, m2 = 2

∴ Hence the co-ordinate of point P is
for point Q,
m1 = 2, m2 = 1

So, the co-ordinate of Q =
Hence, required coordinate of point P and Q is
Question 10.
Find the co-ordinates of point which quarter sects the line joining point (-4, 0) and (0, 6).
Solution :
Let C, D and E are required point which quarter sects the line segment joining the points A(-4, 0) and B(0, 6)

Since D is mid point of A and B, C is is midpoint A and D, and E ¡s mid point of D and B, then AC = CD = DE = EB
Now, co-ordinate of mid point D of A and B.

=
=
Hence, co-ordinate of points divided in four equal part of AB are , (-2, 3) and
, (-2, 3) and  respectively.
respectively.
Find the co-ordinates of point which quarter sects the line joining point (-4, 0) and (0, 6).
Solution :
Let C, D and E are required point which quarter sects the line segment joining the points A(-4, 0) and B(0, 6)

Since D is mid point of A and B, C is is midpoint A and D, and E ¡s mid point of D and B, then AC = CD = DE = EB
Now, co-ordinate of mid point D of A and B.

=
=
Hence, co-ordinate of points divided in four equal part of AB are
Question 11.
Find the ratio in which line 3x + y = 9 divides the line segment which joins points (1, 3) and (2, 7)
Solution :
Let the line 3x + y = 9 divides the line segment joining A(1, 3) and B(2, 7) at point P(x, y) in the ratio λ : 1
x1 = 1 y 1 = 3 m1 = λ
x2 = 2 y2 = 7 m2 = 1

⇒ 6λ + 3 + 7λ + 3 = 9(λ + 1)
⇒ 13λ + 6 = 9λ + 9
⇒ 13λ – 9λ = 9 – 6
⇒ 4λ = 3
⇒ λ =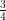
λ : 1 = 3 : 4
Hence, required ratio is 3 : 4.
Find the ratio in which line 3x + y = 9 divides the line segment which joins points (1, 3) and (2, 7)
Solution :
Let the line 3x + y = 9 divides the line segment joining A(1, 3) and B(2, 7) at point P(x, y) in the ratio λ : 1
x1 = 1 y 1 = 3 m1 = λ
x2 = 2 y2 = 7 m2 = 1

⇒ 6λ + 3 + 7λ + 3 = 9(λ + 1)
⇒ 13λ + 6 = 9λ + 9
⇒ 13λ – 9λ = 9 – 6
⇒ 4λ = 3
⇒ λ =
λ : 1 = 3 : 4
Hence, required ratio is 3 : 4.
Question 12.
Find the ratio where point (-3, P), divides internally the line segment which joins points (-5, -4) and (-2, 3). Also find P.
Solution :

Let the point P(-3, p) divides the line segment joining the points A(-5, -4) and B(-2, 3) internally in the ratio m1 : m2.
Hence, x co-ordinate of point P(-3, p)

= -3(m1 + m2) = -2m1 – 5m2
⇒ -3m1 – 3m2 = -2m1 – 5m2
⇒ -3m1 + 2m1 = -5m2 + 3m2
⇒ – m1 = -2m2
⇒ =
= 
Hence required ratio m1 : m2 = 2 : 1
Now, y co-ordinate of point P(-3, p)

Hence, required ratio 2 : 1 and p =
Find the ratio where point (-3, P), divides internally the line segment which joins points (-5, -4) and (-2, 3). Also find P.
Solution :

Let the point P(-3, p) divides the line segment joining the points A(-5, -4) and B(-2, 3) internally in the ratio m1 : m2.
Hence, x co-ordinate of point P(-3, p)

= -3(m1 + m2) = -2m1 – 5m2
⇒ -3m1 – 3m2 = -2m1 – 5m2
⇒ -3m1 + 2m1 = -5m2 + 3m2
⇒ – m1 = -2m2
⇒
Hence required ratio m1 : m2 = 2 : 1
Now, y co-ordinate of point P(-3, p)

Hence, required ratio 2 : 1 and p =







0 Comments