Chapter 2 Real Numbers Ex.2.3 solution.

RBSE Solutions for Class 10 Maths Chapter 2 Real Numbers Ex.2.3 solution.

Class 10 Maths Chapter 2 Real Numbers Ex.2.3 solution. Solution is provided in this post. Here we have provide the solutions of RBSE Boards Books according to chapter wise.

Chapter 2 Real Numbers Ex.2.3 solution.

RBSE Solutions for Class 10 Maths Chapter 2 Real Numbers Ex.2.3 solution.

Question 1.
Prove that 5 – √3 is irrational.
Solution
Let 5 – √3 is a rational number.
we have to find out two integers a and b such as

a, b and 5 all are integers
 is a rational number.
√3 will be also rational number.
But this contradicts the fact that √3 is an irrational number.
So our hypothesis is wrong.
Hence, 5 – √3 is an irrational number.
Hence proved.

Chapter 2 Real Numbers Ex.2.3 solution.

Question 2.
Prove that following numbers are ir-rational
(i)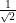 
(ii) 6 + √2
(iii) 3√2
Solution
(i)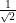  is a rational number
we find two integers b such as  =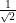 
where a and b co-prime integers (b ≠ 0)
Square both sides

So, b2 divides by 2.
.’. So, b also divides by 2.
Now let b = 2c, where c is any integer
b2 = (2c)2
⇒ b2 = 4c2
⇒ 2a2 = 4c2 (∴ b2 = 2a2)
⇒ a2 = 2c2
Hence, a2, divides by 2.
a also divides by 2.
Hence, 2 is a common factor of a and b.
But, this contradicts the fact that a and b have no common factor other than 1.
This contradiction arises by assuming that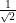  is rational,
Hence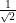  is a irrational number.
Hence proved
(ii) Let 6 + √2 is a rational number
we can find two integers a and b (b ≠ 0)
Such as

a, b and 6 all are integers.
 is a rational numbers
√2 is also a rational number
But this contradicts the fact that √2 is an irrational number.
Our hypothesis is wrong.
Hence, 6 + √2 is an irrational number.
Hence proved.
(iii) Let 3√2 is a rational number we find two integers a and b such as
3√2 =  (where a and b co-prime integers)
⇒ √2 = 
⇒ a, b and 3 are integers.
 is a rational number
√2 is a rational number
3√2 will be also a rational numbers.
But this contradicts the fact that √2 is an irrational number.
So, our hypothesis is wrong.
So, 3√2 is an irrational number.
Hence proved.

Chapter 2 Real Numbers Ex.2.3 solution.

Question 3.
If p and q are a positive prime number then prove that √p + √q is irrational.
Solution
Let √p + √q is a rational number


√q is also a rational number.
But this result is contradicted.
So our hypothesis is wrong.
Hence, √p + √q is an irrational number.
Hence proved.

Hope that the Solutions provided here for Class 10 Maths Chapter 2 Real Numbers Ex.2.3 solution. will help you. If you have any query regarding Rajasthan Board RBSE Class 10 Maths Chapter 2 Real Numbers Ex.2.3 solution drop a comment below and like and share the post.

Thank you.